HAZEL CLIFFORD
A COMPARISON OF GENDER-RELATED ATTITUDES TOWARDS MATHEMATICS BETWEEN GIRLS IN SINGLE-SEX AND CO-EDUCATIONAL SCHOOLS.
Submitted to the University of Exeter for the degree of Master of Education 1998.
This dissertation is a report of an investigation into differences in the attitudes of girls towards the learning of mathematics. It compares two groups of girls at two age levels [grade 7 and 11] taught in a single-sex school with corresponding groups taught in a co-educational school. The focus is purely on attitudinal factors and is not linked to attainment. The purpose is to identify differences or similarities in attitude between girls in co-educational and single-sex education which could possibly impact upon other issues such as confidence in mathematical ability, pursuit of mathematics to higher levels and perceptions of mathematics as an acceptable female choice.
I have researched past and current issues regarding gender inequity [both in general and in terms of mathematical education], the variables which impact upon it and the changes concerning this problem which have been made over the past twenty years in attempts to redress it.
I gathered my information by using a questionnaire to provide results capable of being collated and analysed.
The most significant difference in attitude within this test sample was betwwen the two year 11 groups for the factor of enjoyment. The percentage of girls enjoying mathematics in the single-sex environment was notably higher than their co-educational counterparts [63% v 37% of year group]. This factor alone has many implications, not the least of which is the possible motivation to continue pursuing mathematics to higher levels. For other factors, I have made statistical comparisons and related them to current research.
I have also considered global and cultural aspects of gender inequities in mathematics and the influence of various feminist approaches in attempting to achieve educational and occupational equity.
List of Contents
Outline of problem
Focus of study
An overview of historical and contemporary perspectives
on the issue of gender inequality
Identification of the variables which impact upon it
Global developments in redressing the problem of
gender inequity in mathematics
The influence of feminism on gender-related issues
Single-sex schooling and attitude to mathematics
Research into impacts and benefits of single-sex
teaching on girls� attitudes to mathematics
An examination of research methodology
Discussion of results
Evaluation and conclusions
List of Figures and Tables
Figure 1 Page 19 Differential Effectiveness Model of Classroom Processes
Figure 2 Page 91 Graph to show Attribution of Success [year 7]
Figure 3 Page 91 Graph to show Attribution of Failure [year 7]
Figure 4 Page 94 Graph to show Attribution of Success [year 11]
Figure 5 Page 95 Graph to show Attribution of Failure [year 11]
Figure 6 Page 109 1995 Grade distribution for year 5 BSSC
110 Examinations, by gender
A COMPARISON OF GENDER-RELATED ATTITUDES TOWARDS MATHEMATICS BETWEEN GIRLS IN SINGLE-SEX AND CO-EDUCATIONAL SCHOOLS
The purpose of my dissertation is to make statistical comparisons of gender-related attitudes towards mathematics between girls in a single-sex school and girls in a co-educational school. In conducting this investigation I have considered the recent gender-related alternative conceptions of equity including alternative methods of inquiry and recent developments. Hopefully as studies in new directions evolve, their results will cause presently evolving policies and practices to proceed in a more equitable way.
Although research in this area is an extremely complex process, due to the many factors and their inter-relatedness which impact on attitude and to the inherent problems encountered when measuring such variables as attitude, I am looking for evidence that will test the hypothesis that single-sex schooling for girls provides an environment which enhances girls' attitudes towards mathematics in a positive way. I believe that attitude is the most influential determinant of success or achievement, whether it be in terms of attainment or continued participation, and so this evidence would have important implications.
Previous research in this area has had a major impact on educational opportunity but may have produced equality of opportunity rather than the provision of equity. Much of this research has been evaluated in a quantitative way, perhaps focused on the inputs, processes or outcomes of education, and determining public policy and practice.
According to Secada, Fennema and Adajian [1995], the most recent developments in scholarly enquiry have begun to focus on new areas through research in cognitive psychology, by bringing the problem of gender bias to the fore and seeking solutions.
Globally, education is in a transitional state, where there are frequent contradictory calls for reform. In the U.S., Secada et al.[1995] exemplify these changes by looking at efforts in restructuring the classroom, school or district to efforts that include elements of competition and choice in schooling, which up until recently has been thought of as a free public service available to all.
In attempting to investigate equity, it is necessary to anticipate new social questions and new directions in both research and policy so that equity-based ideas can become an integral part of such policies as they are implemented.
In 1991, the National Centre for Research in Mathematical Sciences Education [NCRMSE] [Secada,G. et al. 1995] commissioned a series of papers from mathematics educators on the education of girls. Among those that considered issues of gender-bias was the work of G. C. Leder, which considers how competent people are disempowered by psychosocial processes in classrooms and this would appear to have great relevance to the issue of single-sex schooling.
This topic is of great interest to me as I have recently joined the staff of a single-sex girls' school - the only surviving single-sex school in Bermuda, where the remaining six government schools and three private secondary schools are all co-educational. As the last remaining single-sex institution, the school has to continually justify why it chooses to remain so, and has an important part to play in educating present and prospective parents, as well as the population in general, about gender inequality and the impact that single-sex schooling can have on addressing this problem.
Analysis of the Problem
According to Carey et al [1994], although gender differences in mathematics achievement have been recognized for almost 50 years, in most cases no special efforts have been made to alleviate them until recently; for example, during the reform movements of the 1960's in which there were major attempts to improve students' learning of mathematics by changing the curriculum, very little attention was given to increasing the achievement of females. Mathematical learning has improved as evidenced by The National Educational Goals Report [1997] which notes that student achievement increased on all mathematics indicators from 1990 to 1996. The report also shows that the US is awarding a higher percent of mathematics and science degrees to all students, as well as to females. Despite this improvement, new and better programmes, in some instances, have allowed existing inequities to be perpetuated, although in a reduced form. According to Carey et al [1994] even the development of a curriculum designed to serve all students has perpetuated inequities. One reason for this is that the developers have not considered what is known about how children learn mathematics with understanding. In some instances there has been little communication between researchers in mainstream mathematics education, who have not been directly concerned with equity issues, and equity researchers, who have not been concerned with critical mainstream research. Before truly equitable classrooms can be developed, concerns about equity and knowledge about children's learning must be integrated. Carey et al [1994] suggest a need for blending research on equity and children's learning, stating that the knowledge gained using a cognitive science research paradigm contributes to our understanding of learning in schools. Research on children's thinking and mathematical concept formation can help inform instruction that addresses gender inequalities. In this way, mathematics education researchers are becoming increasingly well informed about feminist research with mathematics.
In the U.S. there is some evidence that applications for single-sex schooling for girls are rising dramatically. One example of this was cited by Judith Shapiro in her keynote speech for the National Coalition of Girl's Schools' annual meeting [1995]; the increase in applications for Barnard School in the US showed a dramatic increase of over 70% over the past four years. She attributes this rise to the fact that the message advocating single-sex schooling for girls is being heard and more parents are casting a critical eye on co-education and asking just how co-educational these classrooms really are.
She makes a cultural analysis, claiming that America is a nation of poor understanding of social science, with most believing that American society is made up of individuals, and addressing the difficulty Americans have in understanding how society is structured along lines of gender, race, ethnicity and class. She challenges a common perception that if everyone just competes as individuals, success will come to those who deserve it. She suggests that some equity feminists appear to believe that the struggle for gender equity has been won, and that everyone has equal opportunity so there is no need to focus on gender as an issue. Shapiro [1995] points out the naivete of the former remark and the historical improbability of gender equity being solved by one generation for all time. She does not deny the justification for all male institutions, not wishing to deal with the issue of gender asymmetry in single-sex education, but does point out some differences and insists that the purposes and outcomes of all-male institutions need to be compared critically and carefully with the outcomes of all female institutions. Shapiro's opinions, however, are not substantiated through further references to the literature but are included as an illustration of the thinking of some educators supporting single-sex schooling in the US. My research will provide some analysis of this problem.
She discusses what are claimed to be the two major strategies of feminist scholarship, the one emphasizing similarities between women and men, and the other emphasizing differences. The essence of the first approach is to downplay the significance of gender altogether and to argue that gender based discrimination is something that can be overcome once its essential unfairness and irrationality are exposed. The disadvantage of this approach is that it reflects a failure to grasp how pervasive and important a role gender plays in society, and it does women no favour to argue that they should be treated as equals when they are not. The other major feminist strategy argues the existence of important differences between women and men and the goal is to value women for what is distinctive about them and to see that social arrangements reflect women's special needs. The struggle for social change is a struggle to transform a world dominated by men's values into a world more in line with the values of women. Her claim for single-sex girls' schooling is part of the quest for gender equity as an ongoing condition.
Why Gender Differences are Important
There are many reasons why the significant gender differences in mathematics achievement and participation are important. Lucy Sells [1974] was one of the first to argue that mathematics is a critical filter in career choice. Mathematics is increasingly being used in our technological world, and those who opt out of mathematics from 16 onwards will be denied important opportunities. Given the changing job market, many women could be thus disadvantaged in their later lives if they have not pursued the required level of mathematical proficiency. This is perhaps especially significant because a higher proportion of women today need to be wage earners due to changes in family structure and social and role expectations. Many need to be secure in their jobs and financially independent.
Mathematics can also provide the opportunity for a more challenging job which could provide a higher degree of interest and personal fulfillment, on the grounds that it offers more choice of career entry paths and is required to a greater or lesser extent in many professions.
A further significant reason why gender inequities in mathematics are important is that they perpetuate the reproductive cycle of gender inequality in mathematics education [Ernest, 1991], i.e. such inequities will reproduce themselves until halted. This, with other social influences, reinforces gender stereotyping and negatively influences many girls' perceptions of mathematics and their own abilities in mathematics.
Many male students retain the perception that mathematics and science are male domains [Linn, 1990]. The emphasis placed on gender problems in mathematics can itself be counter-productive because it can give credence to the concept that boys are more mathematically orientated. Girls' perceptions of mathematics as a male domain are negatively correlated with mathematical achievement and with taking advanced mathematical courses [Hyde et al., 1990]. Girls who have less sex-stereotyped ideas tend to achieve more mathematically [Armstrong, 1985].
At the college level, the problem becomes more acute; Hewitt and Seymour [1991] found that many women taking mathematical and science-based courses complained that they had to deal with irritating sexist remarks from males on a daily basis, making them feel unwelcome and pressured to achieve. Girls with acceptable academic preparation are choosing careers in these areas in disproportionately low numbers [National Science Foundation, 1990]. In some states the ratio was 3:1 in favour of males choosing college majors in mathematics and science. The National Science Foundation study [1990] also found an alarmingly small percentage of high school seniors who wanted to go into mathematics; the statistics were Girls - 0.5% and Boys - 0.6%.
It appears that middle-class young women and men in U.S. high schools are convinced that they need mathematics. For the first three years of high school gender differences are minimal and efforts to convince girls to proceed mathematically have been successful with middle class girls [mainly by way of convincing parents and counselors] because they see it as a requisite to getting into a good college [Gross, 1988].
Gender differences become apparent at the precalculus and calculus level - the courses needed to major in mathematics, science and engineering [National Science foundation, 1990]. The data referred to here are class- and race-specific. Similar studies on gender differences in mathematics achievement among minority groups are smaller than those among whites [Friedman, 1989]. In minority groups gender differences all but disappear, but this is not a positive advance because with the exception of Asian-Americans, the minority groups score significantly lower than whites and the results indicate that boys and girls score equally poorly.
The problem is not without solution. According to Germaine Greer in "In the Belly of the Beast: Women in Academe", a paper she gave at a conference in King's College, Cambridge in 1993, "there are five times as many female scientists in Latin American countries as there are in Anglo-Saxon" [Pile, 1993,19]. This suggests a strong cultural influence.
Suggested Causes for Differences
There have been many suggestions offered as to why girls perform less well than boys in mathematics. One of these proposed factors is biological differences between the sexes. Various studies have offered explanations for this, but as the achievement gap is closing as women are given more opportunity, many researchers are dismissive of this explanation.
A second proposed factor contributing to gender differences is that of spatial ability. Eddowes [in Burton, 1986:23] and many others claimed that girls' performance in spatial tasks is significantly worse than that of boys. This theory too has been refuted by researchers such as Walden and Walkerdine [1985:23], who examined this assumption and were unable to justify it. Likewise, Walden and Walkerdine concluded that they could not confirm assumptions by Wood [1976], for example, which argue that girls perform better at lower cognitive level mathematical tasks than at higher cognitive level mathematical tasks, and dismiss similar assumptions relating to differing cognitive styles between the sexes.
The factor which I would like to expand on and have chosen to research for my dissertation is that of the difference in attitude and beliefs. There is much evidence supporting the importance of this factor. Griffiths [1992] indicates that research carried out on 750 students at Edinburgh University between 1987 and 1991 showed that female students rated their own IQs lower than those of their fathers and, in three of the five years, higher than those of their mothers. Conversely, male students rated themselves superior to their mothers and, in three of five years, to their fathers too. This suggests a widely accepted belief that men are more intelligent than women. The issue is made worse by the fact that the women being tested, presumably the intellectual elite, should be more aware of gender issues and research, or at the very least, should be more confident of their own ability.
McLeod [1992] identifies three types of component attitudes, emotions and beliefs relating to attitude to mathematics. Firstly, emotions, are intense feelings, either positive or negative, which are evoked by a situation such as being confronted with a mathematical task. Secondly, are attitudes, which are predispositions to act in certain ways given certain concepts, ideas or situations. Attitudes can be held towards mathematics and include [according to Bell et al. 1983] :-
a) liking/disliking of mathematics
b) confidence [or lack of] in own ability
c) anxiety towards mathematics
d) perceived utility of mathematics
Thirdly, there are systems of ideas or beliefs which reflect a person's values and outlook, including beliefs about gender roles and the appropriateness of mathematics for men and women.
Some contend that the importance of attitude towards mathematics is its connection to achievement; for example, Bell et al.[1983] and McLeod, [1992] found a low but significant correlation between these two factors - thus, more positive attitude may produce a higher level of achievement which is further compounded by gender. Research is ambivalent, however, on the attitude - achievement link and there is a much stronger argument that links attitudes to mathematics with future participation.
Perspectives on the Issue of Gender Inequality and an Identification of the Variables which impact upon it
Outline
In this chapter I have attempted to present a very general overview of both historical and contempory perspectives on the issue of gender inequality, to identify the key causal variables which impact upon it and to acknowledge the gendered differences in achievement. I have identified two categories of variables: environmental variables, which include those generated by the school, teacher, peer group, wider society and parents; and learner-related variables, which include cognitive variables such as intelligence and spatial ability, and internal belief variables such as confidence, fear of success, attributions and persistence. Discussion of single-sex education and possible remedies will be dealt with in chapter 4.
For the purpose of this study I would like to distinguish the different meanings of the words "equity" and "equality" as I have found conflicting definitions in other literature. In terms of gendered relationships the implication of the term 'equality of opportunity' is one of fairness or impartiality where an equal chance is being presented to both sexes. The term "equity", on the other hand implies a suggestion of creating an equal state achieved through outcome or circumstance. True equality is achieved only when an equitable outcome occurs, following equality of opportunity.
In attempting to change girls' choices, a deficit view of girls is suggested by many researchers, including Willis [1995], even when the intention is to value and affirm girls. In order to argue disadvantage for girls and justify intervention, differences are often emphasized by reform programmes which target girls, Kenway and Willis [1993], that negatively compare girls to boys i.e. girls lack self esteem or confidence, they fear success, they make poor choices - all relative to boys. In this way, ways of viewing males to females which undermine females are constantly perpetuated. Historically male skills and attributions are seen as the norm against which females become the negative other fostering a general implication that to become equal is to become male.
There is also the issue of implying to girls that if they have the right occupational tools, they will achieve the same job-related success as boys; however, social constraints in the job market block some opportunities and render women worse off in male-dominated fields.
Historical Perspective
Leder [1992] quotes Plato in Book 5 of The Republic arguing that males and females should be educated in the same way for effective career preparation. "There is therefore no function in society which is peculiar to woman as woman or man as man; natural abilities are similarly distributed in each sex, and it is natural for women to share all occupations with men, though in all women will be the weaker partners." [p 295]
Plato may have been the first recorded feminist but his notions, at least on this issue, were almost totally disregarded. Over 2000 years later, Defoe [1697] echoed his thoughts in his anger at society's attitude to the education of women. "I have often thought of it as one of the most barbarous customs in the world that we deny the advantages of learning to women...... If knowledge and understanding had been useless additions to the sex, God Almighty would never have given them the capacity; for He made nothing needless." [Defoe 1697,pp 283-284]
Three centuries later, statistics indicate that in the U.S. and many other Western countries, there are more females pursuing higher education than males [e.g. United States Bureau of the Census,1990],. yet there are many many issues concerning gender-equity which still need to be addressed.
In the 1750s the estimates for literacy in then UK were 64% of adult males and less than 40% of adult females [Lawson and Silver, 1973]. As writing was only taught to reasonably competent readers, and writtten numeracy followed that, the levels of numerical competency would be considerably lower still. During this era it was considered dangerous to educate the working classes and the upper classes usually received a non-utilitarian education which was culturally based either from a governess or at a boarding school where a level of accomplishment in singing, dancing, painting and needlework was emphasized for girls.
The figures for literacy and numeracy rates in the U.S. at this time were typically similar although some regions did boast significantly higher rates in male literacy [e.g. New England, where the rate was 80%]. At this point in history there was little discussion of females' numerical ability; that came later with improved participatory levels in education. It was, in fact, in the 1820s when the school system became more widespread and arithmetic was inserted into the elementary curriculum, introducing it to a higher proportion of girls, that the stereotype of the non-mathematical feminine mind began to evolve. It was from this historical background that today's issues of equality between the genders have arisen. Despite apparent equal opportunities, there are still some subtle differences between male and female participation and achievement in education.
Contemporary Perspectives
Contemporary statistics show much progress towards educational equity, however many small discrepancies prevail. In the UK attitudes of both males and females towards female success in mathematics continue to make significant impact on differences in enrolment for higher level and more intensive mathematics courses and for occupations which require a higher level of mathematical sophistication. Male enrolment thus continues to be significantly higher [Ernest, 1994]. Likewise in Australia, a study by the Department of Employment, Education and Training [1990], provided statistics which showed that males outnumbered females by more than 2:1 in careers demanding tertiary level educational qualifications in science, computing, mathematics and agricultural and vetinary sciences. Less than 25% of managers and administrators were female and less than 10% of craftsmen. On the other hand, more than 60% of salespersons and more than 75% of clerks were female.
Awareness of the gender problem has created many significant changes over the past twenty five years. Much of the focus has been on the achievements of females in mathematics where tremendous strides have been made, in some instances, to remediate discrepancies in attainment in high school mathematics. Rates of attainment however, have not improved proportionately to this. Moreover, able females still lag behind in choice of mathematics courses and mathematics related careers.
Statistics also reveal that despite significant overlap between the genders in mathematical achievement, performance differences on selected mathematical tasks assessed through large scale testing up until 1990 continued to be reported and was usually in favour of males [Leder 1992]. It needs to be considered, however, whether these differences occurred in a "fair field".
According to Campbell [1995], there has been considerable success in closing the gender-gap in mathematics achievement and course taking but the gap persists with choices of mathematics-related college majors and careers. Efforts need to be made to change how mathematics is taught and how girls are treated rather than creating change within girls. In short the population for whom mathematics is appropriate needs to be expanded and redefined.
Identification of variables
Environmental variables
Classrooms, Teachers and Gender Differences in Mathematics
There has been much research examining the influence of the educational environment on the learning of mathematics by males and females. Data gathered from classroom observations suggests that the field is not as fair as formal documents and policies suggest. These observations have revealed marked similarities in the delivery of the lesson, most frequently teacher exposition followed by students' attempts at the work but particular attention has been focused on the ways teachers interact with their male and female students. Brophy and Good [1974] reported that males received more criticism, were praised more frequently for correct answers and had more contact-time with their teachers. More recent work by Gore and Roumagoux [1983] found that teachers gave males longer to respond to questions and Leder's work [1987] showed that males were asked the more cognitively-challenging questions.
The learning environment is made up of many complex factors and is difficult to analyze as many of these factors interact with each other. Apart from the complexity of the impact of the teacher, other variables include texts, materials, physical surroundings and forms of organization. Early research into gender differences in mathematics examined such factors as stereotypic remarks by teachers, use of sex-biased texts and the sex of the teacher. More recently, because of the many types of equity intervention programmes, most teachers are aware of the damage caused by stereotypic comments and avoid using them. Furthermore, in the 1980's, there was an increased awareness of sex-biased mathematical texts leading to a great reduction in blatantly stereotypic portrayals, and Nibelink, Stockdale and Mangru [1986] point out that most texts are now safely non-sexist. Finally, there have been many claims that same-sex teachers incur improved performance by students but Brophy and Good [1985] rejected many of the previous theories offered by researchers in this field. Although a few studies produced minimal conflicting evidence, most showed no differences.
The next level of investigation then focused on the teacher. Many studies including Koehler [1985] revealed the differential treatment received by males that compounded the gender gap. Becker [1981] collected quantitative data using teacher/student interactions and qualitative data using a participant/observer technique in 10 geometry classes for ten days. Her qualitative observations provided a substantial record of differential treatment accorded to males. She found that males received 70% of the encouraging remarks made by teachers, while females received 90% of the the discouraging remarks. Males also received more attention, more acknowledgement, more cognitively challenging questions and more informal interaction.
Stanic and Reyes [1986] carried out an intensive case-study questioning the inequitable treatment of students. They stressed the importance of considering teacher intention when addressing students and also noted that differential student outcomes could be the result of equal teacher treatment, because different students perceive the treatment differently; a teacher remark or behavior that may be helpful to one student, may not be at all beneficial to another. Although there is substantial evidence to indicate that males are treated favorably, there is much less research into the effect that this differential treatment has on achievement.
Differential Effectiveness Studies
Studies at the next level of complexity continued to investigate teacher/student interaction but looked at other contexts such as the types of activities that the students were involved in or their achievement outcomes. These studies were aimed at identifying the classroom processes most effective for teaching either male or female students and are referred to as differential effectiveness studies. Most of the models look like the one illustrated below by Koehler [1985].
Figure 1
Differential Effectiveness Model of Classroom Processes
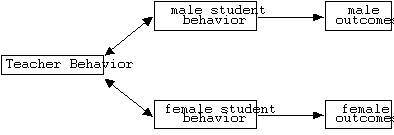
In figure 1, the double headed arrows imply interaction and the single headed arrows imply effect. The figure suggests the different impact of teacher behavior on male and female student behavior and hence the different outcomes by gender of this classroom interaction.
Reyes [1981] conducted a study which examined differential treatment and classroom processes. She found that in some classes the teachers had more interactions with boys, while in others, interactions with females predominated. On examining her findings, she found that the classroom with the predominance of male teacher interactions could be described as having more teacher control. In these classes the teachers had firm control over the class, were aware of what each individual was doing and kept the class actively working on mathematics. These characteristics were described by Good, Grouws and Ebmeier [1983] as being effective teaching practices.
In 1985, Peterson and Fennema conducted a study which examined the teacher/student interaction patterns and related them to mathematical achievement by males and females on different levels of cognitive tasks. Their results were complex and did not produce any clear findings or easy recommendations for teachers to follow. Their only suggestions for teachers to promote higher levels of achievement in females were to give them more opportunity to engage in high-level interactions, to offer them more praise and positive feedback for effort and the use of good strategies, and to encourage them to be independent and divergent thinkers.
Koehler [1985] performed a similar differential effectiveness study in eight algebra classes observing teacher/student interactions and teacher behavior. She found, as with many previous studies, that differential treatment towards students by gender did exist, mainly in favour of males. She assumed that the favored males would perform better than the females; however, her results were not consistent with that assumption. In only one of the six classes in which males were involved in more interactions was the male achievement higher than the female achievement. In two of these six classes, female achievement was in fact higher and in three classes there was little or no difference in performance. In the two classes where females were involved in more teacher interactions, in one females outperformed males and in the other vice-versa. Koehler concluded that there was no clear-cut link between dominance in an interaction pattern and mathematics achievement.
Sex-role Congruency and Mathematics
Teachers, as most other people, have stereotypical beliefs about gender. Sex-role identity influences the cognitive development of males and females in various domains depending on whether or not they see them as an acceptable domain to study for their sex. Evidence suggests that teachers believe that certain subjects are more appropriate for males than females.
A study by Dusek and Joseph [1985] where teachers rated anonymous students with masculine and feminine characteristics on various cognitive traits revealed that students with masculine characteristics were rated higher on intelligence, independence and logic. Mathematics is one subject that is stereotypically masculine and this influences the learning of mathematics by females. Good and Findley [1985] reviewed the literature on sex-role expectations and achievement and concluded that teachers' sex-related beliefs that mathematics is masculine influences their own classroom behavior.
Sex-role Stereotyping of Mathematics-related Characteristics
Fennema et al. [1991] also investigated whether teachers held different beliefs about their best male and best female mathematics students. They used a sex-role stereotype questionnaire containing sixteen personality descriptors that were relevant to achievement in mathematics and four behavioral descriptors [e.g. does not persist or is very persistent in mathematics]. The results showed several significant differences on several items and these were factors of competitiveness, logicalness, adventurousness, loudness, volunteering of answers, enjoyment of mathematics, and independence of mathematics. For all of these descriptive traits, teachers rated their male students as displaying them at a higher level than did females.
Some interesting facts were noted. Initially, the teachers did not strongly stereotype their best male or female mathematics students as masculine or feminine. Only 3 of 16 phrases were seen as differentially describing the best males and females: competitiveness, logicalness, and adventurousness. Secondly, there were significant differences on 3 of the 4 behavioral descriptors; females were seen as volunteering answers to problems less often, enjoying mathematics less, and as being more dependent than males. Thirdly, although males were not seen as more independent overall than females, in mathematics they were. The traits seen as essential to the learning and use of mathematics [competitiveness, logicalness, and adventurousness] were seen as more descriptive of males than females. While avoiding overall sex-stereotyping, the teachers did eventually stereotype their best students in relation to mathematics. Volunteering answers, enjoyment of mathematics, and other overt behaviors were rated as descriptive of males, as was independence, which is deemed as being strongly influential in continued growth in high-cognitive-level mathematics learning.
When examining data such as these, it is important not to overgeneralize and conclude that teachers are overtly biased against females; however there are negative consequences for females caused by what could be interpreted as negative teacher beliefs. The most optimistic explanation of teachers' actions and their implications is that they reflect lack of knowledge. When teachers become empowered by knowledge about gender differences, they will hopefully be able to construct approaches that will diminish the problems.
Teachers' Beliefs and Gender Differences in Mathematics
Fennema [1991] was one of the first to argue that teachers' beliefs and knowledge are important influences on the development of gender differences in mathematics. She states that several teacher beliefs have a profound effect, including expectancies, causal attribution, usefulness of mathematics to both females and males, and gender stereotyping relating to the learning of mathematics.
Identifying and assessing the impact of teacher beliefs provide a very complex task for researchers. They are very often covert, not easily identified, and difficult to measure and study, and some specific teacher beliefs appear to be important influences on the way teachers interact with females and males and organize their classes for instruction.
Different Expectations
Reyes and Stanic [1988] suggest that teachers' attitudes about the "aptitudes of students and the appropriateness of their achieving at a high level in mathematics that differs on the basis of ...... sex" [p 30].,compound the gender difference in mathematics. These attitudes are reflected in the expectations teachers have for male and female learning. Since there has been very little research addressing the problems of differential teacher expectancy for females and males, however, it is difficult to support a conclusion that differential teacher behavior is a reflection of different expectations.
Usefulness of Mathematics
Fennema and Sherman found that gender differences in achievement which favored males were accompanied by a greater male perception of the usefulness of mathematics. Eccles [1983] supported this by his findings that females felt that mathematics was of less value to them than it was to males. If a teacher has a strong belief in the usefulness of mathematics, presumably this has an effect on the students' learning; if a teacher believes that a student's career path will be facilitated by mathematics presumably the teacher will make appropriate choices for the student. There have been some indications about teacher beliefs causing gender differentiation as they tend to choose males over females with the same learning problems for remedial mathematics programmes, suggesting they believe it is more important for males to learn mathematics. Furthermore many teachers actively encourage males to persist in mathematics but do not encourage females. Some all-female schools in Australia have a more limited mathematics curriculum and some co-educational schools have reported scheduling problems that prohibit females from taking advanced mathematics courses. Finally, girls forced to make choices about conflicting advanced courses which include mathematics tend to choose other courses instead of objecting to the conflict [Fennema and Leder, 1990].
Teachers will often encourage females to perform well in routine mathematics and offer them less encouragement to try more cognitively challenging tasks such as problem solving, Expecting also more conformity and dependence from females [Grieb and Easley, 1984]. In Casserly's study [1975] of females in advanced mathematics classes in the secondary schools, Casserly found that many teachers, with good intentions, solved difficult mathematical problems for the girls, fearing tears and negative emotions, thus actually prohibiting the females from becoming successful problem solvers.
Teachers' Perceptions of Students' Attitudes to Mathematics
Attribution Theory [Weiner 1972] identifies a number of perceived causes or explanations of academic success, characterized as internal, external, stable and unstable. These categories may be used to characterize the different attributions of success and failure in terms of specific factors such as ability, task difficulty, effort and luck.
In mathematics participation and achievement for both males and females attributional style interacts with many other internal influences, such as confidence, perception of the usefulness of mathematics and fear of success and greatly influences them. Looking at these components potentially offers a valuable insight into understanding why gender differences in mathematics occur.
Attributions for Success and Failure
Clark and Peterson [1986] researched attributions for the causes of learners' successes and failures in teacher beliefs. They found, "The most important beliefs that teachers have about students are those that deal with the teachers' perceptions of the causes of students' behavior "[p.281] Overt teacher behavior is thus directly related to how the teacher attributes causation of successes and failures .
Studies on teachers' attributions of males' and females' successes and failures have produced varying results. Clark and Peterson [1986] found that the sex of the student was not a variable affecting teachers' attributions. Dweck et al. [1978] reported significantly different evaluative feedback from teachers relating to gender. They found the following for males more than females: positive feedback was addressed to the intellectual quality of the work; less negative feedback was addressed to the intellectual competence of the work; and males' failure tended to be attributed to effort. They concluded that children are reinforced in such a way that males assume their failures are due to insufficient effort and females assume their failures are due to lack of ability. Applying the causal relationship of stable or unstable factors, Dweck et al.'s [1978] results indicate that males are reinforced in a way which encourages them to believe that they can control their own learning; effort is an unstable factor, so if they increase it, they can learn. Females are reinforced in a way which causes them to believe their failures are due to lack of ability and their successes are due to effort; they can only continue to succeed if they exert equal or greater effort in the future. Females thus have little chance to develop confidence in their ability and develop a fear of failure; if they do experience failure they may believe they cannot overcome it. It may be questioned as to whether the damaging effects of teacher beliefs on females about attributions for success and failure would be lessened by removing gender comparisons i.e. by single-sex teaching.
Fennema et al [1991] investigated teacher attributions of their male and female students' learning of mathematics, using thirty eight teachers and their four most able and four least capable students [two males and two females in each case]. They were also asked to select the causes of those students' successes and failures and the following percentage results were obtained: Teachers selected ability as the cause of their capable males' success 58% of the time but only 33% as the cause of their best females' success. Most capable females' successes were due to effort 37% of the time, while capable males' successes were due to effort only 12% of the time. Teachers also said that their least able females' failures were due to ability only 22% of the time. Dweck et al. [1978] reported that teachers made overt attributional statements, reinforcing the perception that males are more likely to succeed because of their ability and females are more likely to succeed because of their effort.
Research evidence suggests that there is a difference in teachers' beliefs about causation and that the more positive belief is held about males. It would be reasonable to assume that such a belief influences teachers as they make instructional decisions.
Classroom Organization
There have been studies performed to examine classroom organization and its effects on male and female performance. Two modes of organizing for instruction are worthy of discussion, namely small group and ability grouping.
Small Group Learning
Most studies on small group learning have not considered the factor of gender: however, two related studies by Webb, [1984] and Webb and Kenderski [1985] involving small groups did consider gender. The samples they used were two above-average eigth-grade mathematics classes and two below- average ninth-grade mathematics classes. Of the small groups involved, some had a majority of males, some had a majority of females and others were equally balanced.
Peer interactions were observed with the following results: in the high achieving classes, the males out-performed the females, the males received more explanations, and female requests for help were ignored twice as often as male requests; females more readily aided their peers; males helped each other more often than they helped females; some females were totally ignored by males especially if there was only one in the group; male questions tended to be much more specific than females'; both sexes asked males to help them more often than they asked females.
There was a marked contrast to these results in the low achieving classes. There were no significant differences in achievement, nor were there gender differences in patterns of interaction. Males did not receive more help and females were equally successful as males at getting their questions answered.
Webb and Kenderski [1985] noted that males were perhaps more persistent than females, since a specific question would indicate that a problem had at least been tackled. In addition males were more secure in that they did not feel compelled to answer others' questions. It is clear that peer interactions within small-group learning could suggest many possible benefits for single-sex schooling; by removing males from small groups, particularly in high achieving groups, females would not be disadvantaged in terms of teacher attention, would be less likely to be misassigned in set placement, and would not be subjected to being ignored by males when requesting help.
Ability Grouping
Hallinan and Sorensen [1987] conducted a study to investigate possible differential effectiveness of ability grouping on males and females. They compared nineteen classes that used ability grouping with twenty nine that did not and their findings were very significant. Not only did they find that there was no differential effect on students' achievement by gender, their results showed that ability grouping for mathematics did not have a direct effect on mathematics achievement in their sample. Their analyses however, did show a gender difference in the accuracy with which students were assigned to their ability groups; they found that the high- ability girls were less likely to be placed in the high- ability sets than were boys, and that girls in general were more likely to be misassigned. This obviously negated the supposed advantage of ability-appropriate instruction especially in high-achieving females and thereby strengthens the case for single-sex groupings.
Recognition of Success Within the Classroom
Rogers [1990] produced a different perspective on classroom interactions. She attempted to find and describe the characteristics of a classroom where females were mathematically successful, and found a North American university with an outstanding record in attracting and retaining students including females, in its undergraduate mathematics program. After many staff and student interviews and classroom observations, she found one particular teacher whose courses were tremendously popular among the students. This teacher made no special effort with regard to female students but did believe mathematical success was attainable by any student prepared to work for it. His methods are noteworthy: he hardly ever lectured and usually allowed students to work in small groups to discuss, argue and negotiate; he would walk around, interject and eventually send a student to the board to illicit further discussion. His teaching success, in short, came about by creating a classroom which was supportive to all students and in which the teaching style mirrored the nature of mathematical enquiry.
The differential effectiveness literature, [Hallinan and Sorensen, 1992] suggests that co-operative mathematics activities might be more beneficial than competitive ones, indicating that teachers should try to produce equity by considering both the quantity and quality of their interactions with students, and that all students should be encouraged to become independent for, previously teachers may have been unwittingly enabling males to become more autonomous than females.
Learner-related variables
Student Variables
Romberg and Carpenter [1986] provide detailed analyses of mathematical content and research that document student strategies for problem solving which are gender-specific; consequently, researchers have developed categories of problem types and models of major levels in children's concept and skill development.
Other researchers concerned with gender differences have studied student behavior and thought processes. In particular the emphasis has been on the affective components of learning, rather than the cognitive aspects other than spatial skills: "The small but recurring differences between females and males in personal-belief variables, such as confidence and risk taking behavior, motivation, and related characteristics - including fear of success, attributional style, learned helplessness, mastery orientation, anxiety, and persistence - continue to attract research attention." [Fennema and Leder, 1990]
Student Attribution of Success and Failure
A further associated factor is the way in which the students themselves attribute causation for success and failure in mathematics. This links with earlier discussion of attribution of success and failure by Clark and Peterson [1986], and Dweck et al [1978]. Wiener [1974] identified four categories to which people attribute their success or failure: ability, effort, task difficulty and luck. These four causes can be classified along the dimensions of stability and locus of control.
These two dimensions of stability and locus of control are important determinants of an individual's future expectations of performance. A student who attributes failure to lack of effort, can adjust this to attain success in the future but a student who attributes failure or lack of success to lack of ability will have very little reason to expect success in the future. On the other hand, a student who attributes success to ability will most likely expect success in the future as ability is a stable factor; conversely, a student who attributes success to luck will hold no such expectation, since luck is outside of one's control.
There have been many studies investigating attribution patterns relating to gender. In 1979, Fennema, Wolleat and Pedro created a Mathematics Attribution Scale which measured attributions for success and failure. They found several gender differences: the males attributed their success to ability more strongly than females, whereas females claimed effort more frequently to be the reason for their success; females also attributed their failure more readily to lack of ability or task difficulty. A comparison between sex and achievement scores showed other gender differences: at all levels of achievement, females were more likely to attribute their success to effort, but as the level of achievement increased, the extent to which they attributed success to effort decreased; for both sexes, the attribution of success to ability increased as achievement increased and the attribution of failure to low ability decreased as achievement increased.
Considering attributions of success and failure in mathematics is particularly interesting because such attributions offer illustration of students' understanding of past events and are also indicators of their future choices; however, the theory is very complex and difficult to interpret but should in time offer great help in understanding gender differences in mathematics.
Confidence
Confidence, which has generally been accepted as a belief about one's competence in mathematics, has been identified as one of the most important affective variables [Reyes, 1984], influencing the students' approach to new material including a determining factor of their persistence. The student will persist if confident of finding a solution or eventually gaining understanding; likewise, a confident student is more likely to participate in mathematical courses at a higher level. Fennema and Sherman [1976] produced the Fennema-Sherman Mathematics Attitude Scales which measured confidence using a confidence subscale; they also measured the students' mathematics achievement. Their results showed that when a gender difference in mathematics achievement in favour of males was found, it was accompanied by a gender difference in confidence, also in favour of males. These gender differences in confidence existed even when there were no differences in achievement.
Leder [1995] states that the weight of evidence in the US suggests that females are less confident than males about their mathematical ability and therefore less likely to persist on difficult tasks. They are also more ambivalent about the value of mathematics as an occupational prerequisite.
Gender differences regarding perceptions in mathematical ability increase as children go through school even when there are no differences in achievement [Educational Testing service, 1988]. Girls in the upper grades like mathematics less, and are less confident doing it. As Fennema and Sherman [1978] found, girls in early adolescence experience a drop in their self-confidence in mathematics before they experience any academic decline. As mentioned previously, this was exemplified by the headmistress of my own school, who in reading the report cards of girls in Years 7 and 8 [ages 11 and 12], found a dramatic decline in self-confidence between two groups of girls, one in Year 7 and one in Year 8; confidence levels in Year 8 were considerably lower in nearly all subjects, including mathematics and science, although academic achievement had been maintained.
Many researchers including Benbow and Stanley [1993], and Fennema and Peterson [1987] claim that the decrease in interest and confidence is probably related to the differential treatment girls and boys receive in school, where parity is apparent but equality is not - i.e. boys interact more often with teachers, material is more appealing to males, boys receive more praise and discipline. This could not, however, be the cause of the lowered self esteem in an all girls school since there were no boys to receive preferential treatment. Many of the girls have brothers who attend a co-educational school, as there are now no single-sex boys schools in Bermuda, and this may be having some impact on the gender stereotyping that occurs within the home, as the boys may be experiencing some form of gender-specific preferential treatment at school, possibly creating the same expectation in the home environment. Moreover, the stereotyping could be a direct reflection of society.
A possible factor which might offer some explanation for the situation mentioned above is the increased awareness by the girls of pressure to succeed from both teachers and parents as this was their first year doing G.C.S.E. work. It was interesting to note that the drop in confidence was apparent in most of the academic subjects. Discussion with Year 7 and Year 8 form teachers suggests that this phenomenon had persisted for many years. On the other hand, the change may be due to adolescence and not gender as other researchers have noted drops in confidence levels of both boys and girls at around age 12.
Usefulness of Mathematics and Fear of Success
The value of mathematics to a female can also be affected by whether or not she considers it an appropriate activity for her sex. An argument for single-sex schooling lies in the self-perceived notion by a female that to succeed in mathematics, she will have to pay a price. In my opinion, an adolescent female is more likely to be influenced by such stereotypical beliefs than is an older counterpart, because of the increased fragility of self-esteem often experienced at this time and the fact that they are constructing their identity as women at this age.
Fear of success also interacts with the following two variables. Horner [1968] identified two sources of negative consequences which impact on many women experiencing success- the individual's loss of her sense of femininity and self esteem, and social rejection because of her success.
When Leder [1982] investigated the relationship between fear of success [FS], mathematics performance, and course-taking intentions for males and females, she found that for high-achieving males, high FS was associated with the intention of leaving school or taking no further mathematics. On the other hand, high-achieving females who were high in FS expressed intentions to take two additional mathematics courses. In terms of performance, she found no relationship between high FS and high mathematical performance for the males. Females who performed well in mathematics were also likely to be high in FS, while for some, being high in FS was incompatible with continued high performance in mathematics. Leder suggests that,, based on her findings, some females reduced their conflict by reducing their level of performance in order to appear less successful or opting out of further mathematics study. Nevertheless, fear of success does not seem to offer a convincing overall explanation for gender differences in mathematics.
The ratio of males and females choosing mathematically related careers has often been seen as a problem relating to girls. Many have assumed the problem to be caused by the girls themselves, either by something they do or by something they lack. In order to solve a problem defined in this way, one can either change the attitudes of the girls or some other impacting influence to make them more compatible with mathematics, or change mathematics so that it is more compatible with girls.
Most efforts thus far have focused on changing girls by encouraging them to use, enjoy, and succeed in mathematics. Efforts made to change mathematics, according to Campbell [1995], have not really focused on changing mathematics but have focused on changing girls' perceptions of mathematics - in reality another version of changing girls. Most of the solutions ignore the role that teachers, schools, and society play in who we are and how and what we learn, by placing the emphasis on changing the girls and not changing society, dooms girls to failure. By changing only one component of a system, only a short term change can be effected; if one creates change in a girl so that she 'loves maths' and then one places her back into the situation that caused her to hate mathematics in the first place, she will most likely revert to hating mathematics.
Other Perspectives
The economic and environmental climate of a country can overshadow initiative to increase females' participation in mathematics. In Australia in particular and to a lesser extent in the U.S., Government's policies of increased retention rates at school occur simultaneously with increased unemployment and decreased job opportunities, causing many young people to become disillusioned and skeptical about the arguments that taking mathematics will increase opportunity for employment: "They are generally able to supply much anecdotal evidence to support their doubts." [Leder, 1992]
Much of the current research on gender inequality tends to concentrate on differences instead of similarities, and this perpetuates the popular stereotypes and beliefs regarding gender differences in mathematics.
Biological Explanations
There have been many attempts to explain mathematical attainment differences by offering biological theories, which have ranged from sex differences related to reproduction, to physiological sex differences which account for certain diseases, illnesses, inherited conditions, and survival rates: for example, as males become more mature at a slower rate, they are more prone to speech defects, vision and reading problems etc; there have also been theories suggesting that a recessive gene for superior visual-spatial ability lies on the X chromosome, thus increasing boys' chances of superior visual-spatial ability; other theories suggest that differences may be traced to prenatal and/or postnatal hormone differences in the lateralization of the cerebral hemispheres of the brain. There is no clear evidence supporting any one of these theories, however, and, as mentioned earlier, most researchers are dismissive of them.
Sex differences in behavioral patterns are influenced by biology; women are more nurturant than men due to hormonal response and there is a great deal of evidence suggesting that males are more aggressive than females. These biological influences may increase the tendency of boys to experience more behavior problems, their greater incidence of learning disabilities, and their greater interest in mathematics and science.
Sex differences in mathematical achievement that are physio-logically influenced can be minimized if teaching methods draw on skills other than visual-spatial skills; the differences are thus negated somewhat when students are taught by same-sex teachers who present material in a way more easily understood by their same-sex students. Another interesting difference to be considered relating to achievement of males and females is the different way they cognitively define and experience achievement or successful accomplishments; Veroff [1977] suggests that males tend to emphasize the impact of their achievement, including what it accomplished and how it compared to the work of others, while females emphasize the process of achievement, including whether or not they accomplished a task alone and if they tried as hard as they could.
According to McLeod, [1988] research on affect has been voluminous but not particularly powerful in influencing the field of mathematics education. He suggests that future research on affect should be linked more closely to the study of cognitive factors in learning so that the affective domain can receive more attention in curriculum development, teacher education, and research on teaching and learning in this field.
Sex Differences in Mathematical Performance and Achievement
There is contradictory evidence from research in this area. According to the APU primary surveys, there was very little difference in the level of mathematical performance with 11 year olds; by contrast, there were significant differences within performance. Walden and Walkerdine [1985] pointed out that boys fared better where spatial ability was required and that the only area where girls experienced a higher rate of success was algebra. During the period 1978-82 the APU, nevertheless, found there to be very little difference in achievement according to gender.
Women's under participation in mathematical courses at a tertiary level of education shows a marked inconsistency with those attaining GCE 'O' levels and GCSE grades A-C [Ernest, 1994]. Although girls' overall results have been higher than those of boys for some time, until recently, boys had consistently outperformed girls in mathematics and science. In 1995, girls equalled boys at 'O' level standard [GCSE] for the first time and, according to Michael Barber, boys will continue to lag behind until the government introduces an intensive programme to improve male literacy at primary school [Electronic Telegraph, 1995]. He stated that the improved achievement of girls, particularly in subjects like mathematics and science was a reflection of a ten year concentrated effort by educationalists to remediate girls' perceived weaknesses; consequently; boys' superiority in these subjects at GCSE level has all but disappeared. This explanation is worth criticising because he overestimates the impact of educational opportunity measures and he neglects economic and social factors which have depressed boys' achievement differentially [especially for the lower socio-economic groups]. In spite of these statistics, women number only 44% of the students in higher education in the U.K. [Ernest, 1994]; in 1989-90 this accounted for a difference of 112,000 persons.
Comparing sex differences in achievement in other countries it would appear that the differences are greatest in the U.S. Most studies agree that girls' greater verbal fluency appears at about age 10-11 and continues through high school and college; likewise, males rise above the national average in math and science at approximately the same time. Some countries, such as Nigeria and England, conducted studies where boys scored higher in reading achievement [Johnson 1973-74], also reporting that both boys and girls in single-sex classes made better academic progress than their counterparts in mixed classes. Germany, Canada and Sweden [Hoiland, 1973] reported the girls-language, boys-math/science difference, but most other European countries showed nonsignificant or inconsistent differences in mathematical achievement.
Leder [1992] states that initial gender stereotypes and their expectations become self-fulfilling, shaped by teachers' as well as students' behaviors, suggesting that much research emphasizes gender differences instead of similarities. Current research methodology needs to be sufficiently flexible to keep abreast of a changing ethos in the classroom and to concentrate on factors which remain inequitable and provide some constructive ways of redressing them.
Global Developments in Redressing the Problem of Gender Inequity in Mathematics
Outline
This chapter investigates gender inequities in other countries and considers the influence of feminism on redressing gender imbalances.
There has been a worldwide shift of the debate on the relationship between mathematics and gender, as a result of feminist scholarship and research on the cultural dependency of approaches, and of performance and participation in mathematics itself. This can be clearly exemplified in the evolution of the SummerMath Program at Mount Holyoke College, which evolved from a summer intervention programme seeking to encourage girls to do more mathematics, to a camp where there is an increased emphasis on encouraging and supporting connected knowing and different ways of teaching mathematics which conform to women's preferred learning styles. This evolution was influenced by feminist theory, in particular 'Women's Ways of Knowing' [Belenky et al,1986], and by constructivist approaches to the learning of mathematics [Kaiser and Rogers, 1995].
There is an increased awareness of the cultural perspectives involved in achieving equity, and doubts exist as to whether models developed in Western countries to achieve gender equity will be effective in all countries, or even with powerless groups within Western countries [Kaiser and Rogers, 1995]. The results of SIMS [Second International Mathematics Study] show in particular that differences in mathematical attainment between countries are larger than those between the sexes [Hanna, 1989]. It also claims that sex-segregated education has been crucial in promoting girls' mathematical achievement.
The Cultural Context
Kaely [1988] compared the cultural influences contributing to gender differences in learning mathematics within different cultures,. observing that the developing world witnesses a greater disparity in the education of both sexes than is apparent in the developed world, where most gender differences favoring boys have disappeared at high school level. He points out the situation in certain matrilineal societies where females achieve the same or better mathematics results than their male counterparts; in the US, Hawaii is the only state in which gender differences in mathematics favour girls. Forbes [1995] examined gender differences in mathematics performance between the two different ethnic groups in New Zealand, finding that the average performance of Maori girls was lower than that of Maori boys, whereas for girls and boys of European origin the average performance was the same. It thus appears that strategies to increase the participation and achievement of girls in mathematics in New Zealand have had a positive impact on girls of European descent, but have not met the needs of Maori girls [Kaiser and Rogers, 1995, p 109].
Leder [1992] examined the influences of print media on gender differences in learning mathematics, comparing two countries, Australia and Canada, and distinguishing among the media images using feminist and societal-psychological lenses. She concluded that the subtle messages conveyed by the popular press are consistent with small but persistent differences in the way males and females value mathematics.
As far as the relationship between gender differences in mathematics performance and enrolment and culture is concerned, Kaeley [1988] suggests four hypotheses which have been substantiated by many other researchers. Firstly, the cultural norms in many developing countries are responsible for producing enrolment disparities; "The women-only environment, which was both reassuring and stimulating, gave women an intellectual legitimacy which enabled them to enter mixed competitive environments such as professional recruitment examinations. Even if they met with less success than their male counterparts, the proportion of women who did achieve success was greater than would be expected given their actual numbers in the preparatory classes" [Imbert, 1994]. Secondly, in the developed world, cultural norms operate to discourage female students in mathematics to the point that their enrolment in mathematics courses declines as soon as the subject becomes optional. Thirdly, in societies where the role of women has changed, gender differences in mathematical performance begin to decrease. Finally, in certain societies and cultural groups in which women have more power and authority, females outperform males in mathematics.
Delon [1993] describes the impact on women's participation in mathematics education after the recent desegregation of the most respected universities in France. Following this action, there was a significant decrease in the numbers of female students studying mathematics and, ultimately, in the numbers of female students pursuing careers in universities, schools, and prestigious professions. The previous single-sex learning environment had been reassuring and stimulating to women and had empowered many of them to enter mixed competitive working environments common in the French society.
Barsksy et al. [1987] reported that France had a higher proportion of female university teachers and researchers in mathematics [24%] than many other countries. The explanation, in part, offered for this is the existence of the ENSs [Ecoles Normales Superieures] from which many of these teachers derive and which were single-sex institutions, the subsequent move to co-education having negative effects on the numbers of women studying mathematics. According to Ferrand, Imbert and Marry [1993], "This amounts to a decline in the number of female mathematicians in these years of over 80%". Previously, females had been allowed to escape the severest forms of competition, and were offered honourable and reasonable professional prospects; with co-education, however, they quickly became the victims of initiation rites and the targets of astonishing rudeness due to their minority status. Before co-education, the ENSs had 5 to 10 times the number of female students than they do today and had produced many of the all too few scientifically renowned female figures, as well as facilitated the establishment of women in the university sphere. The recent decrease is now causing researchers to reassess their present system [Delon, 1995].
Hiddleston [1993] reported on research performed in Malawi where, until recently, there has been very little research on women's issues. In the developing world, in general, there have been very few examples of such research; in the few reported cases, many have been conducted in isolation and difficult to publish, yet over 75% of the world's women live in developing countries. Since research in developed nations cannot be assumed to apply universally, there is a growing body of literature on gender issues in developing countries becoming available; for example, independent research conducted both in Nigeria and in Thailand points to single-sex schooling as providing the most beneficial learning experience for girls [Lee and Lockhead, 1990; Jimenez and Lockhead, 1989].
In Malawi there are three categories of schools: government, which may be either single-sex or co-ed; grant-aided, many of which are single-sex but are still compelled to adopt the selection procedures and charge the same fees as government schools; and private. Statistics from the years 1987-1991 show that in the Malawi Certificate of Education examinations; the percentage of passes in each year was consistently higher in the girls-only schools; the number of girls entering college from the girls-only schools was also substantially higher. The situation in Malawi thus appears to be consistent with that in both Nigeria and Thailand; that is, that girls from single-sex schools meet with greater academic success than their co-educational counterparts.
When making gender comparisons of performance, on average, girls in Malawi are outperformed by boys in all stages of their school careers, and this is particularly acute in the area of mathematics. During the first year of college, however there is a substantial improvement in female performance relative to male; this trend continues throughout year two which reflects a performance at least equal, if not above, to males by the end of the year, and, by year four, female students, are achieving above average in all documented subjects including mathematics and science. Girls account for only 23% of the student population of Chancellor College [one of five colleges in Malawi's only university] despite the fact that they are granted entry to university with lower scores than those of boys and comprise 34% of the university student population as a whole [World Bank, 1988; Chancellor College, 1991]. These findings agree with those of Maritin [1985] whose studies of Kenyan High Schools concluded that girls with lower high school entry grades appear to outperform boys in their final mathematics examinations. In the developed world, however, the majority of research findings contradict these results, showing that male academic performance tends to surpass that of females in early adolescence and this trend continues into later years [ Burton 1990]. Even this trend is now changing, however,in some developed countries like the UK, where girls are beginning to outperform boys in subjects like mathematics and design and technology at 'O' level standard. Meanwhile, girls have outperformed boys in subjects like English for years.
The Influence of Feminism on Gender-related Issues
The influence of feminism on approaches for achieving equity in mathematics is most evident in the area of pedagogy. There is a desire by many feminists to see a fundamental change in the distribution of power in the classroom and consequently in the organization of the discipline of mathematics.
Some contemporary research on gender reform appears to be influenced by some forms of feminism. There are suggestions of how to apply women's ways of knowing, the development of a creative-intuitive pedagogy, curricula changes which are gender inclusive and socially critical, use of independent activities like games at the elementary level, examination of the structures of learning experiences, change in the discipline of mathematics based on philosophical, pedagogical and epistemological questions, an examination of personal experience as the basis of knowledge, and using wider social structures and strategies for intervention.
Redressing the Gender Imbalance in Mathematics
Although there are several differing feminist theories, they share an underlying bond when redressing the gender imbalance in the teaching and learning of mathematics as part of a global project of achieving educational and occupational equity.
Feminists of equality demand legal and actual equality between the sexes and identify the sexual division of labour as the main source of women's oppression. They seek to redress imbalances from an intervention perspective, aimed at increasing the participation of women in mathematics and focus on programmes aimed at resocializing girls. Tobias [1987], illustrates this perspective by focusing on an attitude of the individual student, namely mathematics anxiety, as the source of mathematics avoidance, by assuming that this attitude is learned and therefore changeable [Rogers, 1995].
In contrast, the segregation perspective is often allied to the ideals of feminists of difference, who do not wish to eliminate gender distinctions. It asserts that boys and girls have different ways of learning and that they are better taught separately, using methods and/or curricula appropriate to each. Proponents of the segregation perspective consider it a given, either changeable or worth preserving that gendered differences in learning styles exist. Theoretical general support for this view is found in Belenky et al [1986].
Radical feminism claims neither equality nor difference. Their argument analyses classroom interaction in terms of oppression and dominance and aims at girls' autonomous development without reference to boys. They advocate segregation for other reasons, focusing on the interaction between girls and boys. They argue that in mixed groups, boys always end up getting a greater share of the resources than girls, attributing this gender difference in behavior to socialization. They suggest that changes in socialization, although more desirable, would take too much time and energy in mathematics classes [Morrow C. and J. 1991].
From the perspective that mathematics as a discipline leads to gender imbalance, both radical feminism and feminism of difference show relevance to this view. The former has launched an ambitious project to find androcentrism in all existing knowledge [Mura, 1995]. Feminists of difference may offer the hypothesis that current mathematics is the product of a male way of thinking and speculate about whether 'female mathematics' could exist.
A fourth feminist perspective places responsibility for the gender imbalance on the teaching of mathematics and calls for improved teaching methods that will benefit male and female students alike. All feminist trends have an interest in this perspective. Feminists of difference specify that fairness must acknowledge difference and that equal treatment does not constitute equity. They demand a pedagogy which recognizes women's psychology, is sensitive to student's emotions and is based on collaboration rather than competition. The pedagogy of radical feminists addresses the oppression of women and seeks to minimize hierarchical relations between teachers and students, empowering females and forcing males to relinquish their dominance in the classroom.
Gender imbalance in mathematics is also a political issue. Strategies for redressing it rest on a variety of differing feminist philosophies. By making these strategies explicit, the advantages and dangers of each approach may be evaluated, and prevent the frustration of expecting to share the same attitudes and ideals as other feminists. Because the philosophical and political perspectives are so relevant to the issue, it is difficult to agree on the best strategy for redressing gender imbalance, and it is unlikely that there will ever be a consensus of opinion on the best way to teach mathematics. However, awareness of implicit perspectives contributes to a better understanding of the motivations, aims and effects of the various options and the extent to which they are consistent with our own beliefs and values.
Single-sex Schooling and Attitude to Mathematics
Outline
In this chapter, I examine the views concerning and justifications for single-sex education of various researchers in the U.K. and U.S. I have included initial evidence offered by Dale [1969, 1971, and 1974] through Fennema [1983] to Smith [1986], Stables [1990] and Jacobs [1994].
Changes in Attitude Towards Mathematics from 1970 Onwards
According to Dale [1969, 1970, 1974], the broadly accepted view during the early 1970's was a belief that males were the dominant gender. In society in general, there was an accepted underlying expectation of male authority in which males were in control and defined the terms. The same assumption existed in mixed-sex classrooms; it was undeniable that mixed sex education was considered preparation for 'real life'. Single-sex education, in contrast, was an 'unreal' experience and form of organization because it did not provide girls with a group to whom they were required to defer, nor boys with a group they could dominate. The outcome of Dale's work promoted co-education. He found that co-educational schools were detrimental to girls', but not to boys' academic success, but nevertheless recommended it; his justification was that the social advantages of co-education were considerable and therefore outweighed the depressed academic performance of girls.
For many years, Dale was considered an authority on co-education and his books were very influential. For almost a century, however, feminists had suggested that it was boys who profit from co-education and girls were simply given access to male education which directly and indirectly teaches the inferiority of women [Leder, 1992]. Many of the justifications that were offered for mixed education were later exposed as questionable; for example, it was claimed that girls would have access to male subjects - in reality, due to environmental factors such as timetabling, the opposite occurred. The criticism that single-sex schools delay the process of "coming to terms" with the opposite sex was another misconception; this was, in fact, their success when the terms were so unequal. Other ideas supporting girls' schools claimed that girls were not exposed to bad boy behavior, and, although they were disadvantaged in resources and facilities, there were no guarantees that girls would get their hands on the resources in a mixed school.
By the 1980's, this subject was being pursued by researchers such as Elizabeth Fennema in the U.S. [1980] and others such as Michael Marland in the U.K. Fennema offers the following perspectives on sex differentiation and schooling in relation to success in mathematics.
She first examined the bases of belief that females were not succeeding in mathematics and then provided evidence of schools' effectively changing their female mathematical success rate. She dismissed the argument that because the studying of mathematics was stereotypically male, and because stereotyping of sex roles was so deeply embedded in society, schools were powerless to improve females' studying of mathematics until society changed. She assisted in the development of an intervention programme in the U.S. called "Multiplying Options and Subtracting Bias", the rationale behind which was that simply informing high school females about the importance of mathematics was insufficient. The "Multiplying Options and Subtracting Bias " programme was designed to change the significant groups' [i.e. mathematics teachers, counselors, parents, male students and female students themselves] beliefs about women and mathematics as well as to change each group's behaviour. Initially, nine Midwestern schools were selected to test the videotape series which occurred during the 1978-79 school year and five schools were randomly assigned to be experimental schools. Data collected over the following year formed the baseline from which the amount of change effected by the video intervention was measured, and control schools permitted a comparison of the amount of change in the experimental group to that arising from naturally occurring factors during the two semesters of the investigation. Data were collected on cognitive, affective and behavior variables and showed that changes of greater magnitude were obtained from the experimental group for several variables such as usefulness of maths, maths as a male domain, effectance motivation in maths, information on sex-related differences in mathematics, attribution of success/failure to luck, ability, effort, task and environment, self-report of plans to study high school mathematics, self-report of plans to study after high school mathematics, and school-wide enrolment in mathematics classes. The study used the following instruments to measure their findings:
a]'Fennema-Sherman Attitude Scales': [Instruments designed to measure attitudes towards the learning of mathematics by females and males] Fennema and Sherman [1976].
b] A further scale was developed in the manner of the earlier Fennema-Sherman scales which pertained to this study.
c] 'Mathematics Attribution Scale: an instrument designed to measure students' attributions of causes of their successes and failures in mathematics'. Fennema, Wolleat and Pedro [1979].
Using these instruments, the study of the' Multiplying Options and Subtracting Bias' programme produced many statistically significant positive results amongst both boys and girls, the greater effect being on females - the increase of mathematical course enrolment statistics was greatest among junior year females. Fennema found that the higher the percentage of the school's population involved in the study, the greater the amount of change. It was safely concluded that exposure to the 'Multiplying Options and Subtracting Bias' series did substantially influence students' attitudes about mathematics, increased awareness of the stereotyping in mathematics, and increased students' willingness to take more mathematics courses.
A second intervention programme was also developed, planned and implemented by the San Francisco Bay Area Network for Women in Science [now called the Math/Science Network] [Cronkite and Perl, 1978]. The Network is a unique co-operative effort, the goal of which is to increase young women's participation in mathematical studies and to motivate them to enter careers in science and technology. Subjects were 2215 females who volunteered to attend the conferences. Pre and post conference questionnaires were administered and responses analyzed. The evaluators of the conference concluded that the conference:
1] increased participants' exposure to women in a variety of technical and scientific fields.
2] increased participants' awareness of the importance of taking mathematics and science-related courses.
3] increased participants' plans to take more than two years of high school mathematics. [Cronkite and Perl, 1978]
Both of these intervention programmes described indicate quite clearly that it is possible to change females' mathematical behavior, and to do so in relatively short periods of time.
Comparing the two programmes, the former probably has a more profound effect on the social context of the intervention as a whole, as males were involved in the programmes and hence male awareness of the problem increased. Unless this happens, changes may be reversed or merely moved to a different level of education. The education of boys should not be overlooked by supporters of single-sex girls schools if they wish gender equity in maths truly to penetrate society.
Should the Sexes be Separated?
Marland's work, summarized in Martini [1982], claims that, in certain respects, single-sex schools showed less marked sex stereotyping. In the UK, 16+ and 18+ national examination results showed a proportionately higher take-up and success by girls in the stereotypical subjects of mathematics and physical sciences, and boys in English literature and foreign languages in single-sex schools than in mixed. Similarly subject choice was less stereotypical in single-sex girls' schools than in mixed. [Martini, 1982, p4-6] These differences are consistent with two of the most likely ways in which socialization causes sex stereotyping:
1] Adolescents may develop attitudes which are a reflection of what they guess their peers feel, and accommodate their behavior in ways calculated to win approval from peers. What the opposite sex may seem to think is a great amplifying device; thus, in an all-girls school, there is no reflection from boys that participation in maths has a masculine image. Many researchers have argued persuasively that "the social structures of mixed schools may drive children to make even more sex-stereotyped subject choices, precisely because of the other sex and the pressure to maintain boundaries, distinctiveness and identity" [Marland, 1983].
2] Although teachers of both sexes work in single-sex schools, the chances of women teachers of mathematics and the physical sciences is 50 to 70% higher in girls-only schools than in mixed schools and it is therefore likely that the leadership of strong and successful role models will encourage the pupils towards what would otherwise be non-typical sex efforts and choice [National Coalition of Girls' Schools', Task Force Reports, 1995].
The above speculations are supported by experimental studies by Macoby and Jacklin [1976]. A further influential factor is the issue of learning and teaching styles; the language of the classroom, its topics, its disciplinary style, its methods of encouragement and criticism, and its learning material may be orientated towards boys and thus girls would benefit from separate education where their needs in subjects like mathematics and science can be catered for.
In the UK, the question has to be viewed with respect to the great achievement of schools such as the Girls Public Day School Trust schools which offer remarkably effective academic education to girls. The popular British view is that parents want single-sex education for their daughters, though more will accept a mixed education for their sons. There is probably some relationship here to the historical fact that the most prestigious old foundations in the big cities and among the public schools are inevitably single-sex, thus falsely associating prestige and perceived quality with single-sex education. Likewise, the only single-sex girls' school in Bermuda is regarded by many as the most prestigious on the island.
The reputation of the all-girls, school has been further enhanced by the fact that, in many areas, including Bermuda, where there are mixed and single-sex schools, the balance of the sexes is poor in the mixed schools. In Bermuda, the boy-girl ratio in several government high schools and one of the co-ed private schools is approximately 2:1. In ILEA schools during the 1980s, the mean was 60% boys: 40% girls, with some schools being 70:30 or even more unbalanced [Martini, 1982]. The girls in these schools might thus be disadvantaged by the numerical imbalance.
The argument for single-sex schooling based on the reported academic success of girls-only schools needs cautious interpretation. When the ILEA analyzed its examination results, it found that "when different average ability of intake 'is allowed for' there is no significant difference between examination achievement in the Authority's single-sex and mixed schools" [Martini, 1982]. This investigation clearly showed that apparently better examination results in the ILEA of all-girls' schools can be attributed to the intellectual attainment and social background of the intake of pupils rather than to the organization into single-sex girls' schools.
Conversely, at mixed schools where some single-sex classes for subjects such as mathematics have been organized within the mixed population, some contradictory results were obtained. The first results in the UK of such a scheme for mathematics seemed to follow the direction of single-sex schooling: girls in girls-only classes appeared to do better than girls in parallel mixed classes [Marland, 1983]. An eight form entry school in Tameside put the girls'-only mathematics set in its first year and followed them through, finding that the girls in the single-sex sets achieved a far better average score than girls in the mixed sets and were only slightly below the average score achieved by the boys.
In 1986, Stuart Smith performed research in England to ascertain whether boys and girls were likely to be more successful at maths when taught in a co-ed or single-sex setting. He based his comparison on a group of girls and a group of boys taught in segregated sets for five years [aged 11 - 16] and a group taught in mixed sets at Stanford High School. He divided year 1 into four groups - mixed boys, mixed girls, segregated boys, and segregated girls, matched by ability on a non-verbal reasoning test. In the third year, he tested them using the Tameside Numeracy Test and found that the segregated girls performed significantly better than the mixed girls on 6 of the 13 topics tested, suggesting that, in terms of basic numeracy, the segregated girls had benefited from single-sex teaching. He further found that there was little difference between the two groups of boys. There was also little difference between the overall performance of the mixed boys and mixed girls and that of the segregated boys and segregated girls. When the most difficult items of the test were analyzed separately, however, it was found that the performance of both groups of boys was significantly better than that of the two groups of girls with which they were paired.
In Year 4, the students performed 4 short tests requiring problem solving skills. The overall results of the two boys' and two girls' groups were very similar, suggesting that segregation had little effect on either sex; however, the performance of both groups of boys was significantly better than that of the two groups of girls with which they were paired, differences being greatest on tests of geometry and mensuration.
The pupils also completed the APU Mathematics Attitude Questionnaire at the end of Year 4, which measured the attitude of pupils to mathematics on separate enjoyment, difficulty and utility scales. He found no significant difference in the responses of the two groups of boys to all three of these scales which suggests that the segregated setting had had little effect on the attitudes of boys to mathematics. The group of mixed girls perceived maths as being significantly more useful than their segregated counterparts, but there was little difference in the responses of the two groups of girls to the other two attitude scales. Both groups of girls perceived maths as a much more difficult subject than the two groups of boys. Additionally, segregated boys considered maths to be significantly more useful and more enjoyable than segregated girls. When the boys' and girls' scores were compared, the differences were greater between average and above average ability boys and girls than between below average ability boys and girls, regardless of segregation.
In GCE 'O' level and CSE Maths, the performance of the two boys' groups was very similar and the same was true of the two girls' groups. These results suggested that segregation had had little effect on overall performance of either boys or girls in external maths examinations in this case. Both groups of boys had performed better than both groups of girls although, the differences were not statistically significant.
Interviews were conducted with fifth year girls who found math difficult and it was found that the majority of them believed that their difficulties started between Year 2 and the beginning of Year 4. The girls taught in mixed sets were critical of the boys' behavior, a problem which had an adverse effect on some girls. Nevertheless, the girls mainly attributed their difficulties in maths to the speed at which they went from topic to topic. These girls generally worked in pairs or small groups and there was virtually no contact with boys. Most of the girls who had been in the segregated sets approved of the arrangement but a minority suggested that the mixed set would have been livelier and more competitive. Maths teachers were highly regarded by girls of both groups and assistance from teachers was said to be readily available.
Six full time math teachers were interviewed. All expressed mixed feelings about the single-sex setting, although initial reservations which some felt towards segregation had largely disappeared. They all believed that, in general, girls gained more from a segregated setting than did boys, and that it was more beneficial for younger pupils than for older ones. The majority of these teachers believed that the segregated setting created discussion problems with older pupils, particularly boys. Another disadvantage was that segregation inhibited fine setting and this handicapped the most able boys and girls in particular.
Smith concluded that the results of this investigation suggest that much can be done to improve the performance of girls in maths without recourse to a segregated setting. Nevertheless, it was felt that single-sex sets in the first and second years could be worth encouraging particularly if a special scheme of work designed to meet the needs of girls in maths were developed. It is difficult, however, to generalize from a small local study and because of cultural change, data from the 1970s, 1980s and 1990s have different significance.
Differences in Attitude towards School and Enjoyment of School Subjects by Pupils in Single-sex and Co-ed Settings.
The evidence of Dale [1969, 1971 and 1974] suggests that, generally, both sexes favored mixed schooling for reasons concerning personal happiness and well-being and perceived quality of teaching. The presence of girls was found to be helpful to boys in mixed schools and generally teachers preferred co-ed schools.
In terms of academic achievement, Dale could not find any evidence to favor single-sex schools but he did find a polarization of attitudes between the sexes in mixed schools towards the choice of subjects taken; boys in single-sex schools were more favorably inclined towards stereotypical 'female' subjects such as French, and girls in single-sex schools were more likely to opt for maths and physics.
Ormerod [1975], who investigated preference in the sciences, also noted this tendency, finding a "greater preference of single-sex educated girls and co-educated boys for physics and chemistry and the converse for biology as part of a general phenomenon affecting all subjects". This assertion was not confidently supported by statistics published by the ILEA; however, the ILEA's 1982 statistics did reveal a higher uptake of examination subjects generally by the single-sex educated group of girls and to a lesser extent by the boys.
This bias was not necessarily indicative, however, that single-sex schools provide a superior education, even in academic terms. Steedman [1983] found that mixed and single-sex schools tend to differ in the academic abilities of their intakes. Using evidence from the National Child Development Study which traced the development of 7548 children from birth [in 1958] to age 16, and she found that those pupils who went on to single-sex secondary schools tended to be of a higher social class than those who were co-educated. She concluded that although girls in girls' schools were most likely to pass 5 'O' levels, the majority of girls would not stand to gain at all from single-sex schools.
Results from ILEA contradicted this and indicated that girls do benefit academically from single-sex schooling although the results for boys are less clear [Wilce in TES, 1986]. These results were obtained by an analysis of public examination results in ILEA schools and took into account intake ability.
Bone [1983] dealt with division between the sexes in subject uptake and found that girls do "look more favorably on male areas of study" in single-sex schools, although this was not because girls' schools encouraged this in their options systems. Bone also notes a "special relationship between girls' grammar schools and science" but also found that "in maths and physics girls in girls-only schools do not in general do better than girls in mixed schools". She states that the determining factors in subject choice "are so strong that single-sex or mixed environments can only be expected to modify them and not reverse them" [Bone, 1993].
Opinion poll findings reported by Whitehorn [1984] showed an apparent acceptance by the public at large of the desirability of co-education. Equal Opportunities initiatives such as the GIST [Girls into Science and Technology] project at Manchester have focused efforts on altering attitudes in mixed schools rather than on segregation, although they continued some experimentation on single-sex teaching groups [Whyte, 1986].
A number of researchers are critical of the continued acceptance of co-education. Sutherland [1985] states "There must be few instances where such a radical change in education has occurred in such an absent-minded way" and condemns mixed schools for not providing sufficient managerial positions and hence, role models for women.
The question of girls in mixed schools being alienated from 'male' subjects has long been an issue. Powell and Batters [1986] found that the sex of certain subject teachers makes no difference to girls as long as they have experience of both male and female teachers. Weinekamp et al. [1987] found that girls do suffer from teacher attitudes and behavior in non-traditionally female areas.
The National Curriculum in the UK, by limiting subject choice at age 14 and thus ensuring common core studies, also reduces polarization of subject uptake by gender to some degree. Arguments for single-sex girls schools were given new direction around 1989 by demands from Muslim parents for girls schools [Shaikh and Kelly, 1989].
Stables [1986] performed a study concerned with between schools and between sexes differences in pupils' approaches to third year option choices, third year pupils were chosen as the results would indicate their perceptions of school subjects at the time their subject option choices were being made. From his results, measured using a Likert attitude scale, Stables found notable differences in the subject preferences of pupils in the mixed and single-sex schools; he found a clear preference for language amongst single-sex educated boys and physics was clearly more popular amongst single-sex educated girls. His data did not, however, show any very clear overall preferences for mathematics and the sciences generally among the single-sex educated girls. Overall, the subject preference results supported Ormerod's hypothesis that the polarization of subject interest between the sexes is greater in mixed than in single-sex schools. Ironically, these findings suggest that boys, more than girls, are affected by the presence of the opposite sex in terms of science interest.
In terms of perception of subject importance, Stables found a high degree of agreement between the sexes and between mixed and single-sex schools.
Stables [1990] also examined the differences between pupils from mixed and single-sex schools in their enjoyment of school subjects and in their attitude towards science and school. Although his work in this instance was primarily linked to science, it did provide valuable insights into attitudes towards school and a rank order of favoured subjects with their perceived importance. Over 2300 pupils aged 13 -14 in seven mixed and six single-sex English comprehensive schools were tested on their attitudes to science and school, and were asked to rank in order all their school subjects in terms of liking and of perceived importance. The results of all but the perception of subject importance questionnaire reveal several significant differences between the groups from the mixed and single-sex schools.
Feminist perspectives for redressing the gender imbalance emerge into three strategies, the intervention strategy, the segregation strategy and the teaching strategy. The segregation strategy may be criticized for either reinforcing gender stereotypes by acknowledging that females learn and think differently, or for antagonizing men and increasing tension between the sexes. Historically, sex segregation has not always been implemented with feminist goals in mind, and separate education has often meant inferior education.
Conclusions
These trends can be jointly used to argue that single-sex schooling might be a positive benefit at least during mid-adolescence. It is not surprising that many feminists argue for single-sex schooling given the evidence of male domination in so many aspects of education; however, these data are equivocal and indicate that much depends on classroom processes and teaching, perhaps more so than on the act of separation.
Other Issues Concerning Single-sex Teaching
Progressive educational thinking cannot be assumed to lead automatically to mixed sex education. Some of the arguments put forward about the 'social' benefits seem to be opinionated and unsubstantiated and some measure of single-sex schooling is worthy of consideration despite rejection by particular schools. The introduction of some single-sex classes in an otherwise mixed school is clearly possible; by offering an option of "Mathematics for girls", for example, the message is clearly stated that it is for girls only and it is then possible to use a female teacher and adapt the material and teaching style towards appropriate female expectations. This reassures pupils, and allows for group counseling if required and can use stereotyping in a positive way. Adversely, in some ways, this approach could reinforce stereotyping since it also assumes that all girls and all boys have the same needs and thus the advantages for some may outweigh the disadvantages for others, it masks the real need which is to adapt the teaching rather than polarize the teaching styles.
The debate over which mode of schooling is better has a long history and it is mainly the feminists and those concerned with equity issues who have become involved in the discussions as to the advantages and disadvantages of each form of schooling.
The issue of whether to support single-sex or co-educational schooling is, however, broader than the feminist concern of attempting to help girls study maths, compete equally with boys, or help girls enter university and male occupations. It is an issue which involves analyzing the differences between social class-based practices of education in the state and private sector. In the context of the English state system of education, the most influential modality of class and gender relations has been that exemplified by the private single-sex grammar school, and the introduction in the late 1960s of the mixed comprehensive school in the UK allowed some of its most promising pupils i.e. girls to persistently underachieve [Arnot, 1983].
Support for single-sex education has class connotations in that it is often linked to middle and upper class educational practices. There is support for the idea that co-ed comprehensive schools have more resources to offer a more equal education to boys and girls and will have the facility for bringing to the fore the issue of gender discrimination and prejudice for both male and female pupils and teachers.
Many writers have cast doubt on the overall desirability of co-education, particularly for girls. Data which hint at improved results for girls in single-sex schools are very limited. Around 1985 there were vigorous debates concerning the retention of single-sex schools as part of reorganized secondary provision [Durham, 1985], debates which extend to the possibility of single-sex teaching groups within mixed schools e.g. maths in Years 1 and 2 as already mentioned [Smith, 1986].
Doubts about co-education in the UK exist despite its widespread acceptance and implementation. The results of Stables' study generally confirm those found by Dale [1971], and it is very difficult to evaluate the overall desirability of mixed or single-sex schools.
Stables summarizes the relative merits and demerits of single-sex and co-educational schools in the following statements:
1] His findings confirmed the tendency towards greater polarization of feelings concerning school subjects in mixed schools.
2] Boys feelings are more affected by whether they are in mixed or in single-sex schools than girls'.
3] Girls in mixed schools have relatively more positive attitudes to school than girls in single-sex schools.
The national curriculum does not differentiate between mixed and single-sex schooling, providing equal provision for both sexes but not specifically addressing the issue of differences in attitude between the sexes or between the pupils in mixed and in single-sex schools [DES, 1989]. The danger is that subject interest and specialization may be guided to a greater extent by a desire to conform to a perceived sexual stereotype in mixed schools than in single-sex schools, thus effectively narrowing career choice for co-educated pupils.
An Examination of Research Methodology
When first considering the research methodology concerned with studying gender relations, I anticipated many problems. Mathematics itself is under question, as are the research processes. Each research paradigm has its own assumptions about knowledge, about the the acquisition of knowledge, about society and about education. Thomas Kuhn [1970] first referred to an overall theoretical research perspective as a research paradigm in the context of science, and educational research methodology refers to whole different research outlooks as research paradigms, drawing on Kuhn's notion.
Each educational research paradigm can be said to have three explicit components [Ernest, 1994] which are as follows: the first is ontology, which is a theory of existence concerning the status of the world and what populates it; the second is epistemology which includes the theory of knowledge and the theory of learning; and the third is a methodology which is a theory of application of methods and techniques used to generate and justify knowledge [Ernest, 1994].
An Overview of Research Methodology
Dunne and Johnson [1992] summarized research into gender and mathematics by identifying three dominant strands in research methodology. After characterizing the purposes and methods of each one and describing them, they then explored the conceptualization of gender within each and related them to Habermas' educational interests [1972].
Habermas provided an analytical tool for research by identifying a framework of three "knowledge constitutive interests", - technical, practical and emancipatory. Grundy, [1987] applies Habermas' interests to particular philosophical positions and describes them in the following way : "These interests constitute the three types of science by which knowledge is generated and organized in our society. These three ways of knowing are empirical- analytic, historical-hermeneutic and critical". [Grundy,1987, p10].
Habermas' work provides a powerful analytical tool from a critique position which explicitly constitutes the third interest. The critique position appears to have changed from a biological one, which was quantitative, to critical analysis, which is qualitative, to post modernism. It suggests that the research and the researcher are part of the research environment and influence the outcome of the research; for example, if a feminist were writing the research, sexist research might be influential and would need to be analyzed. This third interest is a position of critique which centres on the absence of an explicit recognition of constitution of knowledge in the technical and practical positions. Lather [1992] uses Habermas' framework and adds her own fourth strand by relating it to post-structuralism
In their review of research into gender and mathematics and science education, Dunne and Johnson [1992] describe a first strand concerned with finding and documenting sex differences using quantitative methods. The focus of this research is on differences in achievement and participation by females, usually gathered through a statistical comparison of male/female enrolment in mathematical courses [Lock, 1992]. The second strand looks for biological differences between the sexes and assumes there are gender differences in aptitudes which have innate causes, attempting to isolate the nature of these differences [Sherman, 1983]. Because of their focus and approach, Habermas' work places both of these strands within technical interests and Lather [1992] links them to positivism. The methodology used assumes the authenticity of the gender categories used, the reliability of scientific descriptions of difference, and the neutrality of knowledge produced. Technical interest research assumes that knowledge is predictable and therefore controllable and unquestionable.
The third strand of research produces social explanations, is more qualitative in nature, and relates to Habermas' practical interest, and the interpretative paradigm. It includes studies which link students' perceptions and attitudes, the influence of the teacher, and sex-stereotyping within the family [Fennema and Leder, 1990].
In looking for ways to solve the problem, a further type of research, broadly labeled interventionist, has arisen [GEMS 1990] which could be included in the strand associated with the critical paradigm, but I am associating it more closely with the practical interest. Some of these studies have attempted to change the learning characteristics of girls so that they more closely resemble those of boys; others have tried to change the curriculum to make it more gender inclusive [Barnes, 1991]. Another set of strategies suggests changes in the learning environment in relation to school organization, such as the implementation of single-sex classes [Burton, 1990].
This has become a fourth strand and is associated with Habermas' emancipatory interest. The research is primarily of a qualitative nature, using interpretative and naturalistic methods. In order to mediate meaning, these research methodologies assume that conclusions produced will adequately describe the social construction of the research site. Their aim is to portray accurately the mathematical learning environment for girls and boys where the effect of the presence of the researcher is assumed to be minimized by the methodology just as it is in the technical interests. It is unrealistic, to some extent, as it denies any form of contamination or disturbance caused by the researcher but most of the current research dealing with gender is in the technical or the practical interests. Habermas' critical interest adds another powerful position to develop research in this field. Walden and Walkerdine [1985] have considered the gender issue more broadly and some of their work has looked at the influence of psychology in education, attempting to understand the role that education plays in the construction of gender.
The Construction of Gender
Dunne and Johnson [1992] took the view that knowledge is produced through social practices and that the knowledge produced is dependent on how the research question is conceived as well as on the processes of the research. Within Habermas' technical interests, the gender categories are considered to be already in existence, of no additional significance and therefore immune to the influence of the researcher. The purpose of the research is to reveal differences which are logical consequences of the existence of gender categories, by objective research methods; the gender differences are thus reflections of innate characteristics of the two sexes.
Research in the third strand, which is qualitative and is associated with Habermas' practical interests, attempts to validate the biological gender differences but emphasizes the social influences in their constitution; the claim is that both biology and social interactions produce the gendered individual.
Interpretative research looks at different experiences and reactions between the sexes and suggests that they may belong to a set of essential gender characteristics. In this area also, the researcher is seen as separate from the research site and although the possibility of contamination is acknowledged, it is considered to be neutralized by the application of triangulation. This research suggests that 'girls prefer collaborative learning environments', 'girls need opportunities to use their language skills', 'girls prefer to share and support each other in tackling mathematical problems', and 'boys perform better in competitive situations than girls'. The remedy for this situation would therefore have to provide girls with sufficient experiences to compensate for their deficiencies [e.g. opportunity to play with spatial toys or assistance in making better choices], or for the teaching of mathematics to address their learning styles [e.g. collaborative work, written work and the use of girl-orientated applications]. The gendered oppositions which 'occur' in mathematics then provide the explanations for interventions. Social constructs such as aggression and compliance or collaboration and competition are then assumed to be characteristics of individuals of each sex. The interventions that are developed from this type of research tend to produce and reproduce the categories they describe; as most classrooms remain competitive and grade-driven, they perpetuate the dominance of masculine traits over feminine traits and reinforce the gender differences by valuing, in terms of mathematical success, such traits as competitiveness, independence and aggression, which are seen in a positive light and are regarded as 'normal', causes the opposing 'female' traits to be viewed negatively.
Consideration of Research Paradigms
In planning this research, I considered the three research paradigms referred to by Ernest [1993] and viewed them with reference to current research into gender and mathematics education and my research problem. The scientific research paradigm intends to produce objective replicable knowledge, generally using statistical, experimental methods which are quantitative in nature such as a structured, predetermined questionnaire, seeking out general laws which can predict future educational outcomes, and examining classroom and learner variables. This paradigm is often associated with the cognitive theory of learning; however it struggles to produce true results as even well confirmed theories are vulnerable to change. Current research on gender in this paradigm, which has its origins in the physical sciences, focuses on differences in achievement and participation by females by collating statistical comparisons of male/female enrolment in mathematical courses, as exemplified by the 3:1 ratio of males to females choosing college majors in mathematics and science [National Science Foundation], 1990].
The interpretative research paradigm developed from the methods used in social science research is concerned with understanding and sense making and uses qualitative forms of enquiry such as case studies or interviews, attempting to negate its subjectivity arising from weaknesses by triangulating multiple viewpoints. The theory of learning central to this paradigm is often a constructivist one, where the mind actively tries to make sense of knowledge gained. The interpretative paradigm uses a particular and concrete instance to suggest, illustrate and illuminate the general case, providing a rich base which allows its readers to identify and empathize with the subjects studied.
Grosz, [1990] offers a criticism of this type of research: "..... in claiming that women's current social roles and positions are the effects of their essence, nature, biology, or universal social position, these theories are guilty of rendering such roles and positions unalterable, necessary, and thus of providing them with a powerful political justification ..... they are necessarily ahistorical; they confuse social relations and fixed attributes; they see fixed attributes as inherent limitations to social change; ....." [Grosz, 1990 p.355]. This and similar statements help to justify a lack of change; however, research within this interpretative paradigm does not necessarily do this.
When considering case study as a research tool, it is necessary to research more broadly in order to establish the typicality of the specific case and to look for shared characteristics with other such cases. When common phenomena have been identified, it is then possible to formulate general summaries and concepts with caution, which could form a broad base for further investigation. There is, however, always a possibility with case study investigations that the material is atypical and therefore subjects or materials need to be critically selected.
The critical-theoretic paradigm resembles the interpretative paradigm but focuses, not only on the knowledge gained, but also on the positive social implications i.e. social change for the better. Current research in the field of gender concerns itself with redressing gender inequalities. Gender research in this paradigm is changed from descriptions based on static categories to more socially dynamic and changing categories [Cornell, 1987], in which the dynamic is brought about by a manifestation of power relationships which are realized through the construction of differences. Gender, in this case, is thus a hierarchical relation produced and reproduced by social practices.
Present educational research on gender and mathematics is most commonly performed in the interpretative research paradigm; methodologies used emphasize the political nature of schooling, usually focusing on social inequities relating to gender, aiming to explain the investments in power which constitute the institutional foundations of knowledge, and stressing that no knowledge is politically neutral.
I will locate my dissertation primarily in the scientific research paradigm by administering a questionnaire to obtain my data. I am hoping that the results obtained will be objective, lend themselves to analysis, and that I will be able to present a systematic record of my findings. When dealing with the issues surrounding gender inequalities, however, I see great potential in each of the other two paradigms. The strength of the interpretative and critical paradigms is in their intended outcomes and commonly associated pedagogical aims where the illuminative outcomes would provide a powerful educational tool in a movement towards gender equity, enhanced by knowledge. I presume there to be a greater interaction or base for applicability of research in the three paradigms in a socially-related area such as gender inequality, than there would be in a subject area such as the teaching of mathematics through an applications approach, where the underlying motive is not so directly linked to achieving social justice.
An advantage of working in the scientific research paradigm is that it can produce precise replicable results and objective generalizations, if the data and methods are robust enough. A weakness of this paradigm is that it ignores the uniqueness of selected variables and assumes that certain factors are entirely fixed or stable. In current gender research, the discipline of mathematics itself is being questioned, along with research processes and the very essence of what it is that constitutes gender. Because of a somewhat insensitive approach to these variations and individual differences, many assumptions drawn from research in this paradigm are regarded as questionable.
Associated work in the interpretative paradigm has the strength of great sensitivity to individuals, their circumstances and their contexts. An example of this is the work of Draper [1989] who examined by interview and case-studies, gender-related attitudes and behaviors as three schools, one co-educatioal and two single-sex, merged into one large co-educational school. I found her work very illuminating and written in such a way that fellow teachers could readily imagine her scenarios and relate to her assumptions; however, the weakness of this paradigm lies in the subjectivity of the enquiry and its results, which are often not open to any form of generalization and therefore the findings are not so readily utilized by other researchers.
The critical research paradigm has the advantage of specifying its goal of improved mathematics education, and therefore does not worry unduly about disturbing its research site. Its basic weakness is that because of hidden institutional sources resistant to change, there is often little or no progress gained from the amount of time invested.
For research in the scientific paradigm to be tenable, certain propositions and assumptions about human behavior have to be made. Key assumptions are made. Firstly, it is assumed that the relationship between variables concerning human behaviour is regulated and predictable. Secondly, it is assumed that these aspects can be observed and measured. According to Hitchcock and Hughes [1992,P 18], "The notion of causality in human affairs suggests that, in fact, human actions can, once correctly observed and identified, be predicted. Ultimately, positivism therefore assumes that there is no qualitative difference between the natural and social world." As positivism developed in the social sciences, two central principles of social research emerged which are worth defining: the principles of 'deductive reasoning' and 'falsifiability' which have become the hallmarks of what is described as scientific method.
Deductive reasoning suggests the possibility of moving from general statements, which can be objective and independent of experience, to particular statements, following scientific research. Karl Popper's book "The Logic of Scientific Discovery" [1959] has been very influential in the philosophy of science. He argues that theories and their subsequent explanations cannot be regarded as being truly scientific, unless they are falsifiable; hence, the main criteria of whether or not a statement has status lies in its testability. This means that researchers working in the scientific paradigm must do two things: they must frame their theories in a way that leaves them open to falsification; and they must be prepared to disregard such theories if they are not open to falsification per se, or they prove wrong in the light of falsifying evidence. Popper's ideas have been highly influential and widely accepted [Hitchcock and Hughes, 1992]. Researchers working in this paradigm use a quantitative approach and are likely to concentrate upon the collection of large amounts of data in order to establish patterns and regularities in that data and test their theories about that data by means of a falsification procedure.
A further term applied to positivistic scientific research is 'nomothetic', an approach which argues that the generalizations or theories emerging from a piece of research must be applicable to a large number of cases or situations. An expression of this is a desire to search for universal general laws but, conversely, biographical, individual or small group studies are seen to be problematic, at least from a statistical perspective.
Criticisms of Positivism and the Scientific Method
Although many researchers choose to investigate within the scientific paradigm, its ontological and epistemological bases have been the focus of sustained and sometimes strong criticism from others. People opposed to positivism and scientific method include some of the best intellectuals in Europe, including philosophers, scientists, social critics and creative artists [Cohen and Manion, 1992]. Essentially, the reaction has been against the world picture projected by scientific research which, it is contended, denigrates life and mind. The actual target of this attack is science's mechanistic and reductionist view of nature which, by definition, excludes notions of choice, freedom, individuality and moral responsibility.
One of the earliest attacks against the scientific method came from the poet, William Blake [Nesfield-Cookson, 1987], who claimed that a mechanistic perspective eliminated the concept of life itself. In this view, quantitative research reduces life to measureable data and no matter how exact measurement can be, it can never give an experience of life, for life itself cannot be weighed or measured on a physical scale.
Kierkegaard [1974] was concerned with the individual and his need to fulfill himself to the highest level of development. The realization of a person's potential was for him the meaning of existence which he saw as "concrete and individual, unique and irreducible, not amenable to conceptualization". He claims that scientific research contributes to the dehumanization of the individual and was very concerned about its objectivity, emphasizing the need to regain subjectivity.
Ions [1977] expresses serious concern at the way quantification and computation are used; "The argument begins when we quantify the process and interpret the human act..... however high-minded the intention, the result is depersonalization, the effects of which can be felt at the level of the individual human being, not simply at the level of culture." His objection is not directed at just quantification but at quantification which becomes an end in itself - a mathematical task rather than a humane study of human condition.
The justification for any research lies in the effect it has on increasing our awareness and degree of consciousness and as the basis for future action. Some researchers, including Holbrook [1977], claim that this has been retarded by the excessive influence research in this paradigm has been allowed to exert on areas of our intellect.
Hampden-Turner [1970], claimed this research to be biased because it is limited and creates an equally limited view of the human being. The results of research are very restricted because researchers concentrate on repetitive, predictable and invariant aspects of their work to the exclusion of subjectivity; this paradigm, thus may not work against any constructivist view of learning because it may fail to take into account man's unique ability to interpret his experiences and represent them to himself.
These are formidable criticisms of the scientific paradigm but alternatives offered by critics produce results which cannot be collated or analyzed.
It is arguable whether gender-equity issues continue to be investigated most effectively through research involving large samples and the application of statistical techniques that are so often used to describe and analyze data thus gathered. By concentrating on group differences rather than similarities, this approach tends to confirm and perpetuate popular stereotypes and beliefs about gender differences in mathematics learning.
Leder [1992] argues that the limitations of attempting to capture and describe the complex classroom environment through any one classroom observation schedule are increasingly being recognized. Conversely, studies that have focused on small-group instruction, on student-student interactions, or on a detailed and intensive observation of small numbers of students have yielded much rich, unexpected, useful and additional information and offer an important perspective to redressing gender inequities in mathematics.
Influence of Feminist Empiricism
Over the past two decades, there has been a growing awareness of the influence that feminist empiricism has had upon research into gender inequities.
According to Harding [1986, 1991, 1993], feminist empiricism begins with the idea that mathematics, science and their global methods are basically sound but that some practices, procedures and assumptions are biased against women. Because these abuses are detrimental to both women and mathematics, they need to be identified and curtailed. The underlying ideas of feminist empiricism are popular with many women mathematicians and have contributed to the problematizing of certain practices within every field of mathematics, a position which has led to detailed analyses of ways in which sexism influences research e.g. Eichler, [1988]. These studies show that gender bias has the potential to affect studies at all levels: in the framing of research problems, in the methods of gathering information, in coding and analyzing data and in interpreting the results. Squire [1989] argues that constructs that have prior masculine associations e.g. aggression, tend to be studied with high-prestige experimental methods, while constructs with feminine associations e.g. anxiety, are studied through the softer methods of questionnaires. Other well used examples of bias include the drawing of conclusions about the general population based upon studies of males and the interpretation of research findings in relation to male norms. Feminist empiricists strive to eliminate all such biases and allow the emergence of new constructs which can provide alternate descriptions and explanations of "the world".
Feminist Empiricism, Gender and Mathematics
Much of the research that has contributed to the study of gender and mathematics belongs to the tradition of feminist empiricism. Early research by Fox, Fennema and Sherman [1977] began by addressing problems in the scientific literature of that time and outlining research issues and agenda for less sexist studies to follow. These early researchers have designed studies and framed questions that avoid many problems identified by Eichler [1988] and Squire [1989] and have introduced many new constructs that have advanced the study of gender, including critical filter [Sells, 1974], mathematics as a male domain [Fennema and Sherman, 1979], math anxiety [Tobias, 1978 and Fennema 1977]. More recently, Fennema and Peterson [1985] have introduced "autonomous learning behaviors," and Turkle [1984] has linked this with "hard" and "soft" forms of mastery; Fennema [1990] has also refined and elaborated the concept of equity. Other researchers have conducted studies of the numerous affective variables concerned with mathematical learning: Reyes and Stanic, [1988] developed psychological models that map the sex differences in the salience and inter relatedness of social and psychological predictors of mathematical achievement; other researchers have challenged sex bias in mathematical testing with some success. In this decade, when tests are used appropriately, there are few sex differences in the measured mathematical performance of the sexes [Linn and Hyde, 1989]. Further, over the past two decades, empirical research on gender and mathematics has reframed the scientific study of women and mathematics. Such work must continue, in order to produce new scientific knowledge and "as a practice of the vigilant critique of "malestream' science" [Damarin, 1995].
Feminist-standpoint Epistemology
"Feminist-standpoint epistemology is a complex approach to the definition and description of a self-consiously feminist way of constructing and conducting science" [Damarin, 1995]. The feminist-standpoint idea was first introduced by Nancy Hartsock [1983] and has its conceptual roots in ideas borrowed from Marxist epistemology. Damarin summarizes these ideas as follows: this is a feminist standpoint which acknowledges that "the world" exists and is knowable through the study of our relations with it. A critical part of that study is interrogating our own position in relation to the objects of that study. The feminist-standpoint idea allows for a multiplicity of truths, which are incomplete, and finds the investigations which begin with the lives of women most valuable, implying a radical shift in underlying assumptions and standards for research on mathematics education. In particular, it requires a willingness to abandon beliefs about the nature of mathematics and how it must be taught and learned in order to be open to the 'nature' of mathematics as it is experienced.
The purposes of feminist research and theory are to understand better the condition of women and to decrease the power of patriarchy in their lives. The status of mathematical knowledge in a society which values technological progress and the mastery of nature, as well as the ascription of mathematical tasks to men, forces feminists to consider and work on the "problem of women and mathematics". In current 'high-tech' society, the ability to understand mathematics is an important aspect of social power; women must therefore claim the right to learn mathematics and to have the mathematical knowledge they have constructed recognized as valuable and acknowledged in curriculum and instruction.
Focus of Study
Having considered the advantages and disadvantages of the three research paradigms, I intend to proceed in the scientific one,.because scientific research is a tool in pursuit of a broader goal; that of gender equity and social justice; however, I may well link my results to research in the other two paradigms if necessary. The main objective of my research is to test the hypothesis that girls in a single-sex institution have a more positive attitude towards mathematics than girls in a co-educational school.
The possible factors which might support the hypothesis and which may impact to a greater or lesser degree on my research are stated below :
1] The impact of differences in achievement between the sexes.
2] Levels of participation in mathematics.
3] Enjoyment of mathematics.
4] Social factors, including the influence of the teacher and sex- stereotyping within the family.
5] Educational practices that seek to neutralize gender inequities e.g. attempts to change the learning characteristics of girls so that they more closely resemble those of boys.
6] The role that education plays in the construction of gender.
7] Learning styles which favour girls.
8] Characteristics of each sex which are socially construed e.g. aggression and compliance.
I will also be considering previous research on factors concerned with the learning environment, namely stereotyped remarks by teachers, use of sex-biased texts, sex of the teacher, differential treatment by the teacher, effects of small group learning and the effect of ability groupings. I intend to consider the impact of the following teacher beliefs which might have significant bearing on gender inequities: expectancies, causal attribution, usefulness of mathematics to both females and males, and gender stereotyping relating to the learning of mathematics.
The Questionnaire
Having decided to proceed with the administration of a questionnaire for my research, although I believe it would be strengthened by other approaches such as interviews or observations, I considered some important aspects when planning it. There is a theoretical justification for including each particular question each of which is intended to be clear, unambiguous, and uniformly workable. I administered the questionnaire myself or used colleagues in order to maximize the response level. I interspersed questions about attitude throughout the questionnaire to allow respondents to air their views rather than merely describe their behavior, and used a 'ticking boxes' technique which is familiar to most respondents. I piloted the questionnaire in a different year group to eliminate ambiguity etc. By having only two administrators of the questionnaire rather than the 6 group teachers, I hoped to minimize differences in terms of completeness, accuracy and uniformity. My questionnaire is designed to ascertain differences in the following aspects of mathematical learning with relation to gender:
a) Students' enjoyment of mathematics.
b) Students' self-concepts in terms of their understanding and ability in mathematics.
c) Students' perceptions of teachers' views of them, [students] relating to ability and effort.
d) Students' estimate of the personal value of mathematics.
e) Whether students enjoy mathematical challenge.
f) Students' confidence in their ability in mathematical tasks.
g) Students' preference for co-educational or single- gender teaching relating to attainment.
h) Students' perceptions of the gendered basis of mathematical ability.
I piloted the questionnaire in my own school on Year 9 students to test how long it took participants to complete it, to check that the instructions were explicit, and to remove any questions which did not yield usable data. I then carried out a preliminary analysis to see whether the wording or format of questions would present any difficulties when the main data are analyzed. I personally administered the questionnaire to the subjects in my own school and used a colleague, who is presently involved in similar research, at the other school. We explained to the students the purpose of the questionnaire and what would happen to the information collected, guaranteeing personal anonymity although the school could be easily identified. As stated previously, the questionnaire was administered to Years 7 and year 11. I hoped the results would provide illustrative evidence of the benefits of single-sex education in the spheres of mathematics. The questionnaire is included as Appendix 1.
Choice of Schools
I realize that any research site in Bermuda is very limited and also unique. Having previously done some research into the gender-problem in a co-educational government school in Bermuda. I am now making comparisons in a single-sex private school and a co-educational private school. As the only all-girls' school in Bermuda is a private one, I used a private co-educational school to make these comparisons. Of only two such schools which exist in Bermuda, one was formerly an all-boys' private school and the other was an academic co-educational government school which became private in 1995. Of the two available choices I chose the latter as the ratio of boys to girls is better balanced. The previous all-boys' school is still only approximately one quarter girls as opposed to nearly 50% in the new private school, and the school which was previously an all-boys school has retained many of its previous teachers which could have significant impact on the way in which the girls are taught.
I started my research by administering to Year 7 students in both schools a questionnaire relating to their perceptions of themselves in mathematics. The sample involved a class at the co-educational school being taught by a female mathematics teacher who is following the Exeter masters program and myself. I then went on to test students in Year 11 [aged 15-16] in order to make comparisons in attitude in mathematics with students four years further into adolescence.
After obtaining results, I intend to examine critically the role of one segregated school in promoting gender equality.
Results
Introduction
In presenting these results, I am making no claims that the group tested in Year 7 will resemble the group tested in Year 11 by the time they reach that age for they are different groups as opposed to a single longitudinal study. The results from the co-ed school might be less typical because the secondary department has only been private for one year. The primary department came into existence as a private school two years ago, obviously drawing pupils from many different educational institutions; at the time of opening, the children were not selected to the primary department according to ability. The secondary department, on becoming private, experienced an atypical shift in student population, magnified by the financial implications of becoming a fee-paying school, and the fact that a few students left to go to other private schools now that their 'free' education was over. The co-educational school had previously been the most academic of the government secondary schools and had selected its intake by ability at age 11 from all the primary schools on the island. It was also the most racially mixed and had an approximately equal number of girls and boys. The secondary department retained this balance when the school became private but the primary department, which included Year 7, although racially balanced, had a much higher proportion of boys than girls. This is possibly due to the fact that one of the three other private schools was a three-form entry girls' school. The gender ratio of this class was twenty boys and five girls, which constitutes a poor representation of co-education but, as a better comparison was unavailable I proceeded with it, realizing my results would not constitute a valid comparison of co-education at this year level.
The all-girls' school has never had an initial intake examination; entry into the school, which is currently in extremely high demand, is based on such factors as date of registration [usually birth], siblings in school, attendance of mother etc. The ability range within the school is, therefore, apparently more diverse. [This factor may or may not affect attitude]. Many of the secondary sample tested had entered the school at age 5 and proceeded through the primary and secondary departments. The school is not as racially balanced as the other, as a much higher proportion of the students are white [approximately 75% in the single-sex school compared to 50% white in the co-ed school]. The year 7 class in, this instance, is also included in the secondary department, which may impact upon attitude associated with teacher expectations/attitude as the co-ed class functions as the top of the primary school and the single-sex class functions as the bottom of the secondary. Both schools are prestigious institutions within the community.
I am focusing mainly on the differences in attitude and not ability between the comparative year groups in the two schools and between the sexes, and then the impact on girls, in the co-ed school. In analyzing these results I have subdivided them into the various attitudinal factors. The numbers shown represent the percentage of students replying in the affirmative. The sizes of the student samples for each year group were as follows:
Year 7 Sample
Subsample
Code: 7ss, female students at a single-sex school in grade 7; n=56
7cg, female students in a co-ed school in grade 7; n=5
7cb, male students in a co-ed school in grade 7; n=20
Year 11
Code: 11ss, female students at a single-sex school in grade 11; n=40
11cg, female students at a co-ed school in grade 11; n=27
11cb, male students at a co-ed school in grade 11; n=31
Questionnaire
The questionnaire used is shown as Appendix 1 and is followed by result tables by school and year group. The questionnaire was adapted from the Girls Into Mathematics' booklet [1985] which in turn was adapted from Barnes, Plaister and Thomas [1984]. The APU [1981] reported that strong, negative feelings were often engendered by the mere mention of the word 'mathematics'; while boys had similar feelings as girls, the APU found that girls were likely to express greater uncertainty about their mathematical abilities and performance whereas boys had greater expectations of success. In order to ascertain in more detail the attitudes of pupils and consider in what ways these might influence mathematical performance, a questionnaire was designed that would illustrate differences in attitude between the sexes.
Comparison of Results by Factor
Enjoyment of Maths
The first factor I am examining is enjoyment of mathematics. In 1981, the APU found that girls enjoyed mathematics less than did boys and part of their dislike was due to the fact that they viewed it as a male subject. Researchers in America and Britain have found that girls tend to see mathematics as 'hard, intellect based and masculine' and that these views sharpen at about the age of 13 or 14+ [Fennema, 1980]; it was likely, therefore, that, the older the pupils, the greater the differences in response.
My results reflect this phenomenon between the pupils at the co-ed school but the single-sex school had substantially different results, indicating a much higher level of enjoyment.
Question 1 related to this factor and obtained the following results:-
1] "I enjoy maths" [Y/N]
Positive responses to question 1
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
n=56 n=5 n=20 n=40 n=27 n=31
1] 70% 100% 85% 63% 37% 77%
Question 1 was a direct question to ascertain the students' stated enjoyment of mathematics. At Year 7 level, 70% of students responded positively to Q1, in the girls' school, compared with 85% of boys and 100% of girls in the co-ed school.
At Year 11 level, 63% of students in the girls' school responded positively to this question, whereas at the co-ed school the numbers were significantly different; only 37% of the female students enjoyed maths compared with 77% of the males, which may imply that the single-sex education of girls preserves the enjoyment of a subject otherwise seen as a largely male domain.
In considering factors which may have contributed to these positive results, particularly in the co-ed school at Year 7, one which is worthy of mention is the quality of the teaching. I have observed the Year 7 teacher using co-operative groupwork, organizing seasonal projects and outings incorporating mathematics, both of which were generally accepted with great enthusiasm and interest, facilitating the attribution of the high levels of enjoyment experienced by the students partially to the teachers' personal approach. I also need to add a note of caution concerning the tiny sample size which means generalisation is unwarranted for the Year 7 co-ed girls.
These results support the findings of the APU [Joffe and Foxman, 1988] who carried out extensive testing on attitudes and mathematics in the 1980s. They found that, at age 11, girls and boys enjoyed maths almost equally [girls slightly more] but that by age 15 boys enjoy maths significantly more. From from my results, however, girls at age 15 at the single-sex school still enjoyed mathematics significantly more than their co-ed counterparts [63%-37%], although this still was lower than the male figures of 77%. If these results were replicated for the same groups of students by a longitudinal study, it could indicate that single-sex teaching contributes to the retention and fostering of a more positive attitude towards mathematics; however, I am unable to conclude this from my results of different groups.
14] "I think my maths teacher enjoys teaching me" [Y/N]
Positive responses to question 14
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
14] 84% 80% 85% 68% 81% 68%
Q14 was an indication of the students' perception of teacher enjoyment. At Year 7 level, 84% of girls in the single-sex school responded positively as did 80% of girls and 85% of boys in the co-ed school. At Year 11, level 68% of girls' replies in the single-sex school were positive and in the co-ed school 81% of girls and 68% of boys were so. In this sample, co-ed girls in Year 11 have a significantly more positive perception of teacher enjoyment. There is no obvious reason for this disparity.
Q 13 - "I do not like it if I miss a maths lesson" [Y/N]
This was an attempted indication of enjoyment/confidence and motivation, although the interaction between these variables could be interpreted in so many ways that little significance can be attached to the results. For example, students may reply positively because they are confident that that they could quickly catch up on any new work presented or they may reply positively because they lack confidence in their ability to comprehend the new work anyway so missing a lesson would have little impact. There is also the option of the student who is not motivated sufficiently to care whether they attend every lesson.
Question 13 turned out to be a poor question but this was not apparent to me after the pilot testing. The results in the pilot test showed little discrepancy from my expectation, so at that point I did not make any further interpretation. It would, however, have more value as an interview question when further clarification could be sought by the interviewer.
Positive responses to question 13
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
13] 48% 20% 55% 45% 56% 16%
The figures here are very difficult to interpret. Their greatest difference was between the sexes at Year 11 level; only 16% of boys said they minded missing a math lesson, whereas 45% of single-sex girl and 56% of co-ed girls did not like to miss lessons. These figures could be indicative of boys' higher confidence at maths or they could reflect lack of motivation or even bravado. I think they can only be considered when linked to other questions. At Year 7 level there was also a considerable difference between the sexes [35%].The co-ed girls were much less concerned about missing a lesson [20%] compared with the single-sex girls [48%] or the co-ed boys [55%]. It is very hard to interpret this data beyond speculation.
Q11 "I enjoy trying to solve a new maths problem" [Y/N]
This is also a reflection of the students' enjoyment of mathematics where confidence and the challenging nature of mathematics are also incorporated into the question. The question elicited the following percentages:
Positive responses to question 11
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
11] 52% 60% 70% 40% 26% 52%
The results show an expected difference in all three categories at the Year 11 level, with the boys' figures being higher in both age groups. There was little difference between the two girls groups at Year 7 level [8%] favoring co-ed girls, and this was reversed in the Year 11 level [14%], indicating that the single-sex girls displayed slightly more confidence in their ability. These figures are broadly illustrative of research indicating that boys are more confident in their ability to solve problems and that single-sex schooling for girls might help to negate decline in confidence levels for problem solving.
Perception that ability in maths is innate
Q2 - "You have to be clever to do well at maths" [Y/N.] examines the view of mathematical ability. The historical or traditional view of mathematics is that it is a very specialized branch of knowledge and belongs to those able to pursue it at a high cognitive level.
Positive responses to question 2
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
2] 23% 0% 30% 30% 41% 61%
The differences in responses here are quite dramatic. I speculate that the lower percentages of positive responses in the younger students are indicative of the fact that mathematics is a more approachable and acceptable subject to them; students of this age might thus find that mathematics is more appropriate and contextual. Perhaps the level of mathematics lends itself more readily to social and cultural activities and investigations and therefore their concept of it as a hierarchical discipline is lower. Measuring and comparing student height statistics for example is much less "pure" than solving problems by applying algebraic formulae. This might explain why the Year 7 figures were lower; perhaps they are less aware that their tasks are mathematically based. Also they [7 cg] are a small, possibly unrepresentative group.
The perceptions of the older students show tremendous differences in responses. In dealing with a higher level of mathematics, the perception that you have to be clever to succeed at it is increased, indicating that their perception of mathematics is more fixed and less attainable by those who do not have a cognitive grasp of it. One factor with obvious implied consequences in my results is the perception of the co-ed Yr 11 boys where 61% thought being clever was intrinsic to success at mathematics. In comparing this reply to other questions, it is worth noting that this same group had the following response to their own perceptions and their teachers' perceptions of their ability in maths:
Question 10 "I believe that I am good at math" [Y/N]
Question 7 "I think that my math teacher thinks that I am good at math" [Y/N], provided the following results-
Positive responses to questions 10 and 7
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
10] 79% 80% 95% 58% 37% 74%
7] 84% 100% 95% 48% 37% 74%
10] Co-ed Yr 11 girls positive responses 37%-self perception
7] 37%-perception of teacher's view
10] Co-ed Yr 11 boys positive responses 74%-self perception
7] 74%-perception of teacher's view
The boys' high level of positive response to the initial question [2] may be somewhat linked to their perception of their own math ability and their self esteem and therefore have more positive implications. If their perception of mathematical success is linked to a positive perception of ability, it will obviously increase their self esteem. Conversely, the much lower girls' responses to the linked questions [10 & 7] could indicate that girls' perception of their ability is lower, but the low response to the original question indicates that girls do not perceive a certain cognitive level as a requirement for success in mathematics.
The differences between the Year 11 girls at the single-sex school and the co-ed students - girls [11% difference] and boys - [31% difference] is also significant. Girls in the single-sex school have a different perception of the ability to succeed in mathematics, notably that it can be achieved by effort and desire and is therefore more readily attainable.
The differing perceptions possibly reinforce the myth that mathematical ability is fixed and male. The large difference in female response between the two year levels at the co-ed school [41%] compared to the equivalent difference in response in the single-sex school [7%] would suggest negative implications for girls being taught in a co-ed setting. It might indicate that exposure to boys at secondary level in maths lessons increases the stereotypical gender belief that maths is a male domain. Girls in the single-sex setting thus remained relatively unchanged in their perception that success in math was available to them.
Attribution of Success and Failure
Questions 3,4,15,and 16 relate to the attribution theory and locus of causality for success and failure. Using the Attribution Theory of Weiner [1972], described in chapter 1, who identified the following categories to characterize the differing attributions of success and failure namely - ability, task difficulty, effort and luck - the following results were obtained:
Q15] "If I do well in maths it is usually because":
[ ] I am naturally good at it.
[ ] I work very hard.
[ ] I was lucky
[ ] The work is very easy.
Year 7 Success
ssg g/co-ed b/co-ed
skill and ability 16% 0% 20%
hard work 70% 60% 70%
luck 11% 20% 5%
low task difficulty 8% 20% 0%
Figure 2 Graph to Show Attribution of Success - Year 7
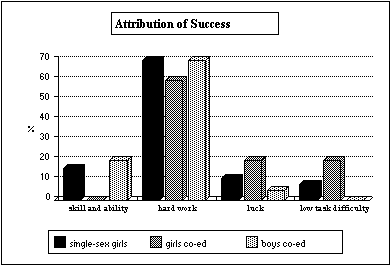
Failure
ssg g/co-ed b/co-ed
lack of skill and ab. 15% 20% 0%
lack of hard work 75% 40% 75%
bad luck 5% 20% 5%
task difficulty 5% 20% 15%
Figure 3 Graph to Show Attribution of Failure - Year 7
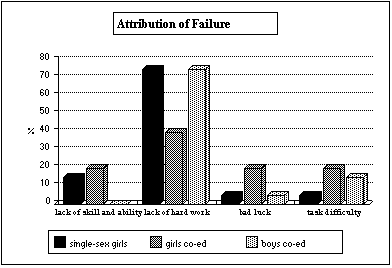
The most striking difference here is the similarity, particularly in attributions of hard work and luck, between the girls in the single-sex school and the boys in the co-ed school and the difference between these two groups and the girls in the co-ed group.
For success - hard work - 10% difference
luck - 9-15% difference in favour of the girls in the co-ed school
For failure - lack of hard work - 35% [difference between the single-sex girls and co-ed boys compared to co-ed girls] and, likewise, bad luck - 15% difference
For hard work attributed to success and lack of it attributed to failure, the percentages were amazingly identical. At Year 7 level, 70-75% of single-sex girls and co-ed boys attributed their success/failures directly to hard work or lack of it. According to Ernest [1994], more boys tend to explain their success in terms of internal and stable factors such as skill and ability, where more girls typically attribute their success at maths to good luck, study efforts or good teaching and their failures to lack of skill and ability. My results indicate very different beliefs and contrasts with many research findings. The percentages of single-sex girls attributing success/failure to luck or lack of it were extremely low [11% and 5%]; the boys' results for this factor were more comparable [5% and 5%]. A more significant difference occurred between the single-sex girls [11% and 5%] and the co-ed girls [20% and 20%] although these percentages are still relatively low for their sex', suggesting a possible move towards the elimination of this single difference between the sexes at this level.
The attribution of hard work to success or lack of it to failure showed a similar pattern within the three groups. The single-sex girls' and co-ed boys' percentages were high and identical [70% and 70%, = success due to hard work, 75% and 75%, = failure due to lack of it]. The difference between the two female groups was more pronounced, particularly the latter [70% and 60% = hard work and 74% and 40% = lack of it]. The results favoured the attribution of success to hard work of girls in a single-sex school over their co-ed counterparts although both were higher than recent research had led me to believe. Attribution to task difficulty was also low in all three groups - [low task difficulty 8% ss girls, 20% co-ed girls, 0% co-ed boys and task difficulty 5% ss girls, 20% co-ed girls and 15% co-ed boys]. In my testing at this level, the percentages in the single-sex girls' results are extremely low [8% attributed low difficulty as a cause for success and 5% attributed difficulty as a cause for failure]. The boys results are similarly and expectedly low [0% low difficulty for success and 15% difficulty for failure]. The results of the co-ed girls [20% for both] were favourable for their sex but not as extreme as the single-sex girls, - differences being [8% -20% low task difficulty/success and 5% -20% task difficulty/failure]. These results contradict the general pattern of research in this area and demonstrate very little gender difference for these factors.
At Year 11, the following results were obtained.
Attributions for Success
caused by:
ssg g/co-ed b/co-ed
-skill and ability 17% 11% 42%
-hard work 63% 40% 36%
-luck 17% 20% 6%
-low task difficulty 3% 30% 16%
Figure 4 - Graph to Show Attribution of Success - Year 11
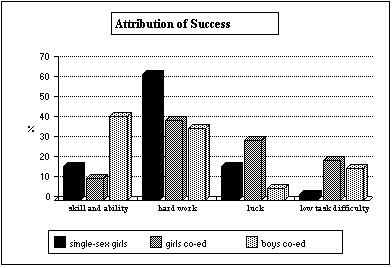
Attributions for Failure
caused by:
ssg g/co-ed b/co-ed
-lack of skill and ab. 15% 11% 0%
-lack of hard work 57% 59% 75%
-bad luck 3% 4% 6%
-task difficulty 25% 26% 16%
Figure 5 Graph to Show Attribution of Failure - Year 11
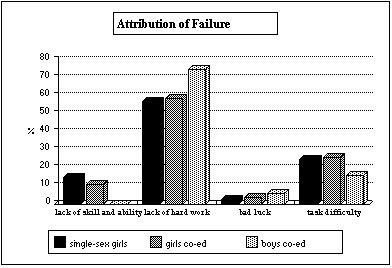
In this sample, too, although the attributions were slightly more diverse, the girls' results were similar to each other, and different from those of the boys. The boys' results were the most diverse: although 42% of them attributed skill and ability as a cause for success, 0% attributed lack of skill and ability as a cause for failure; 75% of them thought that lack of hard work was a cause of failure, but only 36% thought that success could be attributed to effort. The girls' attribution to natural ability contributing to success was markedly lower [girls ss 17%, girls co-ed 11%] than that of the boys [42%] and would obviously impact on their confidence. 63% of single-sex girls attributed hard work to success, whereas only 40% of co-ed girls did, this number being much closer to that of that of the co-ed boys. The trend was similar for lack of hard work being attributed to failure, but this time the girls' percentages were much more similar [ss g 57%, co-ed g 59% and co-ed boys 75%].
The attribution of success to luck and failure to bad luck was low for both single-sex girls [17% and 3%] and co-ed girls [15% and 4%] and was not very different from the boys'. According to Ernest [1994], the girls' results do not follow the pattern of recent research, but when compared to similar findings at the Year 7 level, might indicate other factors are impacting on the girls' beliefs. Consistent with these findings is the lower attribution to task difficulty; in the UK and USA, girls typically attribute success to low task demand or difficulty or good teaching [Ernest, 1994], but my results would indicate otherwise, particularly with single-sex girls. Single-sex girls' results were lower [3%] than both those of the boys [16%] and those of the co-ed girls [30%]. This, too, reflects the trend of the Year 7 single-sex girls, where low task difficulty was only 8%. These results would indicate that girls in this single-sex school do not believe that lack of task difficulty is responsible for their success in maths. For task difficulty attributed to failure the girls' results were still lower than previous research would suggest [25% - ss girls, 26% - co-ed girls] compared to those of the boys [16%] but showed no difference between those of the two girls' groups.
These results were confirmed for the test samples by similar results for questions 3 and 4, these being:
3] "You have to work hard to do well at maths" [Y/N]
4] "I am lucky when I do well on a maths test" [Y/N]
My results were as follows:-
Positive responses to questions 3 and 4
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
3] 91% 80% 90% 85% 100% 84%
4] 39% 80% 10% 30% 59% 23%
The boys' response to question 3 was typically high [90% - year 7 and 84% year 11] but the girls' responses were equally high [91% and 85% ss and 80% and 100% co-ed], confirming the differences found by questions 15 and 16 when compared to recent research. The co-ed Year 11 girls' result [100%] suggests a very different perception of attribution, namely that hard work is a requirement of success.
For question 4, the results followed the same atypical pattern, although to a much lesser extent: [Yr 7 ssg 39% and boys 10%, and Yr 11 ssg 30% and boys 23%] - but, remarkably, the co-ed girls replied in a more typical way [80% and 59%]. These results were not very consistent with their previous responses unless they consider good test results to be a combination of both hard work and luck. The single-sex girls' results were notably different and suggest an attitude more closely resembling that of boys.
Question 4 indicated an important difference between single-sex girls and co-ed girls; the percentages of co-ed girls attributing luck as a possible reason for doing well on a maths test was approximately double that of the single-sex girls at both year levels [39% ssg, 80% co-ed g at year 7, and 30% ssg, 59% co-ed g at year 11]. The results of the co-ed girls' group were more consistent with previous research but the single-sex girls indicated a change in belief that was closer to that of the boys in this sample.
Q19 is a further measure of the students, perception of their natural ability. In this instance, their replies can be affected by their individual effort and to some extent is a measure of their confidence. It could also be a response to the usual [expected] task demand i.e. task difficulty.
19] "When the teacher asks another student to answer"
[] I usually know the right answer
[] I sometimes know the right answer
[] I hardly ever know the right answer
The students replied as follows:-
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
Usually 37% 0% 45% 28% 3% 29%
Sometimes 63% 100% 50% 65% 78% 71%
Hardly ever 0% 0% 5% 7% 19% 0%
This question also illustrated important differences between the single-sex girls and the co-ed girls. Expectedly, the highest percentages of positive replies were in the 'sometimes' category across the board. The most significant difference was in the co-ed girls' groups at both levels. For Year 7 level, all the replies were in the 'sometimes' category; at Year 11 level, only 3% placed themselves in the 'usually' group and 19% opted for 'hardly ever'. This contrasted with the other two groups which were quite similar - single-sex girls and co-ed boys ['usually' 28% and 29% and 'hardly ever' 7% and 0%]. These figures support the hypothesis that girls in a single-sex school have much more confidence in their ability or are more comfortable with their expectation of task difficulty.
Effect of the Presence of Boys on Learning Environment
Q20 is a reflection of the atmosphere in the classroom and is aimed at discerning whether or not the co-ed girls felt that the presence of boys disrupted the learning environment.
Q20 "In lessons most people work hard":-
[] All of the time
[] Most of the time
[] Some waste too much time
The following results were obtained:-
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
All 25% 0% 25% 2% 7% 3%
Most 70% 80% 60% 55% 45% 45%
Waste 5% 20% 15% 43% 48% 52%
The figures reveal a higher percentage of students perceived to be applying themselves at the younger age level which, I speculate, could probably be expanded to include many other subjects, although caution must be exercised because of the small sample.. The Year 11 figures, on the other hand, indicated very little discrepancy between all three groups, the percentages roughly falling into reasonably similar groups feeling that either most people worked hard or wasted too much time. I had decided not to compare sets within a school but know from my results, as the questionnaires were administered in ability-grouped mathematics lessons, that most of the 'most' category replies came from the higher sets at Year 11 level and most of the 'waste' category replies came from the lower Year 11 sets in both schools. Year 7 sets in both schools were mixed ability so I was unable to make any assumptions here. This factor had much more impact on response than the type of school which in this sample, had virtually nil. Further, there was no evidence of any belief that boys in a co-ed setting caused disruption in the lesson. A further explanation of the lack of significant differences between the two girls groups could be that the girls in the single-sex setting had taken on the disruptive or attention seeking role of the boys and negated their impact upon their statistic.
Confidence
Many researchers including Bell at al. [1983], have examined the gendered differences in attitude components such as confidence and the impact they have on attainment, enjoyment, and pursuit of mathematics, and anxiety towards it, which will also relate to their attribution of success or failure. Research findings , in general, express the view that girls rate their own ability lower than males at higher levels of education and hence they demonstrate a lower level of confidence. Hanna Beloff's [1992] study on university students, for example, illustrates this.
Question 12 asks for a direct response to the question of confidence in maths.
12] "I feel confident about my ability in maths" [Y/N.]
The results were as follows:-
Yr 7 Yr 11
ssg coed g coed b ssg coed g coed b 77% 60% 70% 45% 33% 65%
An initial examination of the results indicates that the single-sex girls' confidence levels were higher than that of the co-ed girls. In fact, at Year 7 level, the single-sex girls' result was higher than that of the boys [77% ssg, 70% co-ed boys] with co-ed girls shown to be the least confident; however, the differences are relatively small. At Year 11 level, the co-ed girls were the notably least confident group [now only 33%]; the single-sex girls were only 45% - significantly less than the Year 7 statistic, but still considerably higher than that of their co-ed counterparts, and the male percentages remained largely unchanged [70%-65%]. These results are illustrative of recent gendered research but also show slightly raised levels of confidence, as is claimed by advocates of single-sex schooling, in single-sex girls over the co-ed girls [Yr 7 ss 77%, co-ed 60%, Yr 11 ss 45%, co-ed 33%].
Q 10 "I believe that I am good at maths" [Y/N], is a self perception of ability which might be correlated with confidence. My questionnaire provided the following results:-
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
10] 79% 80% 95% 58% 37% 74%
These results correspond to those of question 12, replicate the general trend at Year 11 level, and illustrate the typical difference in confidence of the girls at the two year levels. For this question, the co-ed boys' confidence was 95% at Year 7 level, higher than the girls', and there was minimal difference between the two girls' groups. At Year 11 level, the boys' confidence was 74%, while the girls' results were 58%-single-sex girls and 37% co-ed girls, a further possible illustration of girls' confidence relatively worsening with age but the single-sex one remaining higher than that of the co-ed girls.
Q 5 "I usually understand a new maths idea quickly Y/N obtained the following results":-
Positive responses to question 5
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
5] 66% 100% 80% 33% 52% 61%
It is a further indication of student belief where student perception of ability to grasp a new concept quickly can be directly related to confidence. These results show a reversed pattern between the two girls' groups both at Year 7 and Year 11 levels where the co-ed girls have a more confident belief that they can grasp new concepts quickly [Yr 7 ss 66%, co-ed 100%, Yr 11 ss 33%, co-ed 52%]. In fact, the Year 7 co-ed girls appeared to have a higher level of confidence than did their male counterparts and, even at Year 11 level, the percentage difference was only 9% [52-61] compared to the expected lower girls' score of 33% in single-sex girls. This result may be interpreted as meaning that single-sex girls have less confidence in their ability to grasp new concepts than do co-ed students, but it could also mean that they are given more difficult work to master.
In comparing the two questions with opposing results as far as the two female groups are concerned, an analogous interpretation is suggested; one hypothesis could be that the single-sex girls consider themselves to be more competent at applying knowledge, once knowledge is assimilated and the co-ed girls consider themselves more competent at grasping new concepts initially. This theory is supported by the attribution rate results which indicate that single-sex girls attribute their success to hard work more so than do the co-ed girls where hard work may be seen as persistence at a task to promote understanding and/or practice to conceptualize it.
Both year level results are indicative of the belief that girls' confidence decreases with age and in these samples the boys' confidence, too, was considerably lower in the older group.
Perceived Usefulness of Maths
Differential career expectation is a further contributory factor which may help to shape the differences in attitude and hence performance differences between the sexes. Girls' expectancies of mathematics becoming an integral factor in their future education is lower than that of boys who often exhibit more interest in the subject due to the nature of their career plans. Questions 8 and 9 pertained to the students' perceptions of the usefulness of maths to their future endeavours. The questions were as follows:-
8] "Knowing math will help me get a job" [Y/N].
9] "I think math will be an important part of my job when I leave school" [Y/N].
The results I obtained were as follows:-
Positive responses to questions 8 and 9
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
8]96% 100% 95% 88% 81% 90%
9]84% 60% 85% 63% 63% 68%
At Year 7 level the perceived usefulness of maths was extremely high throughout all three groups [96%, 100% and 95%], demonstrating no noticeable difference either between the sexes or the schools. These figures can evoke no significant comment from me other than perhaps an indication of a positive move towards gender equity within this small sample, in that girls now also think they need mathematics for work. Question 9 at this level showed a parity in the percentages of single-sex girls and co-ed boys [84% and 85%] with co-ed girls considerably lower at 60%. The co-ed girls group showed the most marked difference [40%] in the utility of maths for getting a job and maintaining maths at the job. For the latter factor, this was 24% below the single-sex girls' indicating that math was viewed as a lesser factor in their career plans at this stage. The other two groups showed 12%[ssg] and 10%[boys] differences.
At the Year 11 level, the single-sex girls' results closely resembled the boys' for Q8 and the co-ed girls'' for Q9. All three scores were high for the perceived utility of maths [88%-ssg, 81% co-ed g, 90% boys] and dropped at similar rates [63%, 63% and 68%] for question 9. The girls' scores here were identical - both being only 5% lower than the boys. These figures contradict recent research but give a very positive reflection of efforts being made to close the gender gap.
Such results link easily to questions 17 and 24 which examine the perception of the importance of ability in math between the sexes.
17] "To be good at math is":
[] More important for girls
[] More important for boys
[] Equally important for both the sexes
The results were as follows:-
Positive responses to question 17
17] Yr 7 Yr 11
girls boys equal girls boys equal
ssg 4% 0% 96% 5% 3% 92%
co-ed g 0% 0% 100% 0% 11% 89%
co-ed b 0% 0% 100% 0% 6% 94%
The results in all six categories overwhelmingly supported the belief that being good at mathematics is equally important to both sexes -another encouraged result in the campaign for gender equity. It would appear from my results that this is no longer a factor in gender inequality as everyone now recognizes the importance of mathematics; at year 7 level the reply was almost unanimous with only one girl from the single-sex school believing that maths ability is more important for girls.
At Year 11 level, the trend was basically the same with the majority of students believing maths to be equally important to both sexes. The discrepancies here were slightly larger but still particularly low; a small percentage [5] of girls at the single-sex school believed math to be more important for girls, and 3% considered it more important for boys. Noticeably, at the co-ed school no-one thought maths to be more important for girls, but 11% of girls and 6% of boys deemed it to be more important for boys. In fact, the only replies which favoured girls were from the single-sex school.
Q24 was very similar but worded differently:-
"Do you think it is more important for -"
[] Boys to be good at maths?
[] Girls to be good at maths?
[] It is equally important for both.
The results were as follows:-
Positive responses to question 24
24] Yr 7 Yr 11
girls boys equal girls boys equal
ssg 7% 0% 93% 5% 3% 92%
co-ed g 0% 0% 100% 0% 7% 93%
co-ed b 0% 0% 100% 3% 0% 97%
The results were expectedly consistent with those of Q17 where the majority of students opted for math being equally important for both sexes and again the single-sex girls' sample had a minimal perception of maths being more important for girls. None of the co-ed girls deemed maths to be more important for girls, but at the Year 11 level 7% of them thought it to be more important for boys. The only other variant was at Year 11 level where 3% of boys thought maths to be more important for girls.
Natural Ability
Q23 attempts to ascertain whether the students believed that one sex possessed more natural ability than the other. Past research indicates a strong belief that maths is a male domain but many educators have pursued the elimination of this belief.
Q23" Which sex do you think is naturally better at maths?"
[] Boys
[] Girls
[] Both sexes do equally well.
Positive responses to question 23
23] Yr 7 Yr 11
girls boys equal girls boys equal
ssg 7% 0% 93% 15% 20% 65%
co-ed g 0% 20% 80% 0% 7% 93%
co-ed b 10% 15% 75% 3% 0% 97%
Comparing the two groups of girls, although the widespread perception was that natural math ability was equally distributed, the only females who thought that girls' ability was higher attended the single-sex school [Yr 7 7%, Yr 11 15%]. More co-ed boys, in fact, credited girls with higher natural ability than did the co-ed girls [10% level 7 and 3% level 11- compared to 0%, 0%].
Conversely the single-sex Yr 11 group had the most diverse results , 20% favouring boys, 15% girls, and only 65% thinking natural ability was equally distributed. It is important for gender equity for boys to have this perception of ability being equally distributed. In this sample, the Year 11 result was so high [97%] that it contradicts the findings of the attribution theory of Weiner [1972], but offers much hope to those concerned with gender equity.
Teacher Attention
Questions 18 and 21 relate to the students' perception of the distribution of teacher attention. Traditionally, boys have monopolized more than their share of teacher attention time and this fact has long fuelled a loud message from advocates for single-sex schooling.
Q18 "The teacher usually asks me to answer:"
a] [] As often as anyone else
b] [] More than anyone else
c] [] Less than anyone else
Positive responses to question 18
18] Yr 7 Yr 11
a b c a b c
ssg 70% 2% 28% 88% 12% 0%
co-ed g 80% 0% 20% 63% 0% 37%
co-ed b 75% 0% 25% 81% 0% 19%
At Year 7 level the perceptions were fairly evenly distributed with the majority of students feeling that they had their fair share of teacher attention. Although the results were broadly similar, the biggest difference [10%] was between single-sex girls [70%] and co-ed girls, which contradicts the appeal for single-sex schooling. At both year levels, the only students who thought they received more attention were at the single-sex school but the numbers were low [2% at yr 7, 12% at yr 11].
At Year 11 level, the results more closely resembled the outcomes of current research; the single-sex girls positive percentage was even higher than the co-ed boys' [88% - 81%] and these were clearly higher than the co-ed girls' [63%]. In considering the b] and c] responses, the single-sex girls' responses were further removed from both co-ed groups. The remaining 12% of single-sex girls thought that they had the teacher's attention more than did their peers whereas the remaining 19% of co-ed boys thought that they had their teacher's attention less than did their classmates. The remaining 37% of co-ed girls also indicated they believed they had their teacher's attention less than did their peers. If these numbers were combined, the results would be more dramatic in favour of the single-sex girls' group and positively exemplify one of the main claims for single-sex teaching.
The following table illustrates the results of combining the first two options which would constitute a positive category in question response [teacher asks as often or more] and compares it to the negative response [teacher asks less].
Combined results Year 11
ss co-ed g co-ed b
Teacher asks as often or more 100% 63% 81%
Teacher asks less 0% 37% 19%
This combination of responses reinforces the perceived differences in teacher attention between the single-sex girls and the co-ed girls. The single-sex girls' response was 100% in the positive category [teacher asks as often or more] compared to only 63% of co-ed girls.
Q21 examines the teacher attention factor but from a broader angle, and it includes aspects such as individualized help when students are working. The results for this question were as follows:-
21] "Do you feel you have the teacher's attention":
[ ] More than most other students?
[ ] Less than most other students?
[ ] The teacher's attention is equally distributed.
Positive responses to question 21
Yr 7 Yr 11
more less equal more less equal
ssg 3% 26% 71% 5% 22% 73%
co-ed g 20% 60% 20% 7% 45% 48%
co-ed b 10% 25% 65% 9% 9% 82%
For this question, a similar pattern became apparent at Year 7 level as well as at Year 11. As I expected, the single-sex girls' and co-ed boys' responses indicated a more positive perception of teacher attention. In Year 7, the percentages of more and equal in the single-sex girls' group and co-ed boys' group were comparable [ss-74% co-ed b-75%] and much higher than those of the co-ed girls [40%]. In seeking a possible alternate explanation for the low percentage from the co-ed girls, I looked at questions regarding their perceptions of their ability in case their thinking was that they required less attention from their teacher because they were more able, but comparing their beliefs to those of the other two groups, this seems implausible.
The results at Year 11 also generally confirm the belief that single-sex schooling for girls produces a higher incidence of teacher attention for them; here, the percentages were 91% for more or equal for the boys' group and 78% and 52% in the two girls' groups [ss/co-ed g]. Mahoney [1985] cites the male dominance of teacher attention time as one of the most important influences on pupils' level of attainment, reinforcing girls' feelings of inferiority and neglect. In both year level samples, the number of co-ed girls who considered that they had less teacher attention time was more than double that of the single-sex groups.
Student perception of single-sex v co-ed teaching.
Q22 examines the students' beliefs about whether or not they would learn better in a single-sex or a co-ed class.
Q22 "Do you think you would learn more if your class was"
[] Boys and girls together?
[] Just your own sex?
Positive responses to question 22
Year 7 Year 11
ss g co-ed co-ed b ss g co-ed g co-ed b
Boys & Girls 20% 60% 100% 5% 81% 78%
Own sex 80% 40% 0% 95% 19% 19%
The results here were very polarized with the vast majority of students supportive of their own environment. I think 'ownership' of the school may be extremely influential here - certainly at the single-sex school, students hear the message 'loud and clear' and school events are frequently used to reinforce the positive benefits of single-sex schooling. The differing beliefs between the two female groups are even more pronounced at Year 11 level with single-sex-95% and co-ed-81% for their own form of schooling. One possible positive implication from these results is that it would appear that most of the students are satisfied with their type of schooling.
Student perception of teacher attitude
Q6 and 7 sought to examine the student perception of their teacher's perception of their effort and ability. Q14 examined the student perception of their teacher's enjoyment of teaching them and was also considered earlier.
Q6 "I think that my maths teacher thinks that I work hard at maths" Y/N.
Positive responses to question 6
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
Yes 80% 60% 90% 63% 63% 55%
No 20% 40% 5% 37% 37% 45%
At Year 7 level, the percentage for single-sex girls somewhat resembled that for co-ed boys [80%, 90%], while the co-ed girls' was considerably lower [60%]; this factor could be influenced by lower confidence or self-esteem in the co-ed girls group. At Year 11 level, the figures are very different; the single-sex and co-ed girls' groups were identical at 63% and the co-ed boys' was lower at 55%. This has several implications: firstly, the students may themselves think that they are not working so hard, perhaps with a lesser need for teacher approval due to their age; secondly, the lower scores for the boys may be a reflection of personal confidence in their ability and hence a lesser need to work hard. It is very difficult, however, to make comparisons as the personality of the teacher is such a variable. These results might reflect much of the child's own perception about effort as they would believe that a teacher would recognize hard work; however, recognition of hard work by a teacher has significant impact on motivating the student to continue her/his efforts.
In order to make comparisons about Bermudian females' overall success compared to that of boys, I tried to obtain data from the other three private schools; however, none of them was willing to release its results so my only comparisons are with students in the eight government schools; I then made comparisons of grades obtained by Year 5 students in 1995 in the Bermuda Secondary Schools Certificate examinations which involved 210 boys and 231 girls, the numbers presented in the tables indicating the percentage of the students, by gender, who achieved the stated grades, firstly within the exam group and secondly within the year group. The results demonstrate the range of BSSC mathematics examinations: the highest academic level is the Traditional examination which is based on the University of London GCE, syllabus B; the next level is the Commercial level, which is less academic and focuses on business mathematics with the inclusion of some algebra and geometry; the third level is the least academic and is designed for the least able students. This data is the only other measurement of attainment in mathematics in Bermuda available to me, but constitute statistics available from a different type of school i.e. government schools as opposed to the private schools where my data were collected.
Figure 6, 1995 Grade Distribution for Year 5 BSSC Examinations by Gender
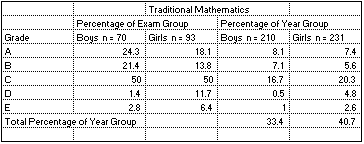
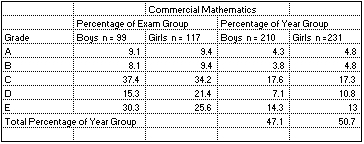
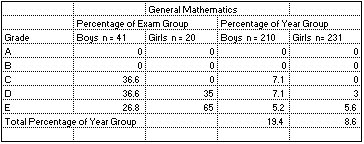
These figures indicate that slightly more boys achieve the highest grades, and these figures illustrated in the tables and my own experience lead me to believe that top sets have a higher proportion of girls. Further, the bottom end of the general table indicates a higher percentage of boys within that category, although the boys in this instance attained slightly higher grades than did the girls. It is very difficult to relate these figures in a meaningful way to my own data but they are included as the only illustrative Bermuda data on attainment available to me.
Q7 "I think that my maths teacher thinks that I am good at maths" Y/N. is also very difficult to analyze but provided the following results:-
Positive responses to question 7
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
7] 84% 100% 95% 48% 37% 74%
This question, too, is possibly largely impacted by the students' own perception of self and confidence. The Year 7 positive responses were very high in all 3 groups [84%, 100% and 95%, the lowest score being the ssg] and probably reflect a very positive attitude of both their ability and their teacher. The Year 11 group reflected the research findings of girls losing self esteem at this age. It would be interesting to compare the figures with ability ratings to dismiss this as being a true reflection of ability. The boys, score was much higher [74%] than both of the girls' although the single-sex girls did fare more positively than did the co-ed girls [48%, 37%]. This female perception of lowered belief in ability is one of the critical issues in girls pursuing maths at the next level. From this result it would appear that single-sex schooling might have had a small effect on girls retaining a positive attitude towards their mathematical ability, but that perhaps broader social influences are dominant here.
Q14 pertains more to the student perception of the climate in the classroom and the value attached to the students' learning needs.
Q14 "I think my maths teacher enjoys teaching me" Y/N.
Results
Year 7 Year 11
ss g co-ed g co-ed b ss g co-ed g co-ed b
14] 84% 80% 85% 68% 81% 68%
It was encouraging to see such a positive response, particularly at Year 7 level, where the results are more or less the same for all three groups. The co-ed girls' response was somewhat higher than those of the other two groups at Year 11 level which would indicate an absence of significant gender differences between the younger groups and a more positive attitude towards perception of teacher enjoyment to teaching themselves by the older co-ed girls; however, such results could also mean co-ed girls are offered less criticism which is needed for learning.
Summary
In making comparisons between the two test samples, I found the following possible indications of benefit to girls from attending a single-sex school in this case. Several questions showed varying responses from the two female groups and in many cases the response by the single-sex girls was almost identical to that of the boys and in a few cases was higher. Generally, there were fewer distinguishable differences in attitude between the younger students and more pronounced ones at the Year 11 level, which fits with international studies.
A very significant difference was the degree of enjoyment at Year 11 level between the single-sex girls [63%] and the co-ed girls [37%]. I believe enjoyment of a subject is so important that it is an educational goal in itself. As the student sample from each school was the total year group [i.e. four different teachers ] in each case, and both schools follow the same basic curriculum, I ruled out these factors as being the main cause of difference; thus differences in the make-up of the sample other than by gender could have been a possible cause of difference. This factor alone impacts on so many others, not the least of which is that of motivation to pursue mathematics at a higher level, that it should be addressed when considering the two different classroom environments. The difference was also reflected in the question pertaining to the challenge of solving a new maths problem, where again, the attitude of the single-sex girls was more positive at year 11 level [ss-40%, cg-26%]. In equating success to ability, the single-sex girls at year 11 showed a lower percentage of positive replies [30%, 41%].
When considering attribution theory, the single-sex girls at Year 7 level almost replicated those of boys, particularly with the attributions of hard work and luck to success. For one question at Year 11 level, the results were diverse and the previous pattern had disappeared; however, there were significant differences at both year levels when considering the factor of luck. Many more of the co-ed girls attributed their success to luck; the single-sex girls, on the other hand, exhibited a strong tendency to attribute their success to natural ability and effort, i.e. internal, stable factors. The single-sex girls were more confident at both levels and showed a stronger belief in their own ability at Year 11 level.
In looking at teacher attention, the single-sex Year 11 girls perceived they had their teacher's attention as often or more than did their classmates, while 37% of the co-ed girls perceived they had less attention. At Year 7 level the single-sex students had a demonstrably higher perception of their teacher's perception of their effort and ability.
Conversely, the co-ed girls perceived themselves as being quicker to grasp new concepts, a result which is strangely inconsistent with the overall trends in their answers. The questions relating to student perception of teacher enjoyment and student displeasure of missing mathematics lessons were also inconsistent with the co-ed girls' previous responses.
Regarding student perception of the most appropriate type of schooling, each group showed strong support for its own learning environment.
The Year 11 girls also had a higher perception of their teacher's enjoyment of teaching them. This factor could possibly be directly related to the individual teacher but, at this year level both schools have four sets for mathematics with different teachers, which would reduce the impact on the statistic which any one of them might generate.
Factors which did not seem to be influenced by the type of school in this instance were:
1] students' perception of teacher enjoyment
2] students' dislike of missing lessons
3] presence of boys causing disruption in the classroom
4] perceived usefulness of maths to obtain job
5] perceived usefulness of maths within job
6] perceived importance of ability to each sex
7] perceived equal distribution of natural ability between the sexes
8] Year 11 teachers' perception of students' perception of effort and ability
For factors 4 and 5, the positive responses were very high and for factors 6 and 7 they were overwhelmingly so. It would appear from this sample that some positive steps have been taken in the movement towards gender equity.
Chapter 7 Evaluation and Conclusions
My test sample of two schools was so small that little significance, for general purposes, can be attached to the results. In particular the number of Year 7 co-ed girls is tiny, [n = 5], so the Year 7 results must be viewed with special caution. Moreover, the sample and test site were so unique that I am unable to generalize from my results even within the islands of Bermuda. A further factor which could have influenced my results is the fact that girls rather than boys will admit to feelings of anxiety and lack of confidence, and in this way, thus gender differences perpetuate gender stereotyping.
In Bermuda, the difference in standards of educational opportunity and practice between the private and government schools are dramatic and continues to widen, a fact illustrated by the entrance demands now being placed on the private schools which are higher than they have ever been. It is difficult, therefore to make valid comparisons of achievement data between the government and private schools, as their educational background is so diverse but, unfortunately this was the only information available to me. In addition my selection of schools was extremely limited and the two chosen schools may not be that comparable, a weakness in my testing which I could not improve upon. It is important for me to stress this point: because the two schools amount essentially to an opportunity sample, it is not possible to claim that they are perfectly matched. Therefore some of the differences could be due to random variations between the school populations. This fact must be borne in mind when considering the outcomes of this study and the differences revealed.
Furthermore, although the global evidence from limited data demonstrating the benefits of single-sex schooling is neither wholly convincing nor without contradiction, the results of my own enquiry have nevertheless strengthened my belief that it has a valuable place, at some point, in the mathematical education of girls. It is my belief that it is, in fact, possible and beneficial to both sexes to have a mixture of both single-sex and co-educational schooling for optimum results in terms of both social and educational goals including those of mathematics. Such a system would include segregation at some point during early primary and again during early secondary years. I would suggest a co-educational preschool situation allowing social interaction. This would be followed by segregation during early primary years when behaviour differences between the sexes seem more pronounced. After further co-education throughout the primary years, I would suggest segregation during the first two or three years of secondary education when girls are at their most fragile emotionally and forming their own identities, and boys lag behind somewhat in terms of physical and emotional development. After this second period of segregation, I would advocate all further education to be co-educational. This would be an interesting topic to pursue for further study.
My results lend themselves to three pairs of comparisons. The first is between the groups of single-sex girls and co-educated girls. The factors which show the greatest difference in this group are enjoyment at Year 11 level, where the single-sex girls demonstrate a much higher level of enjoyment; attribution of luck to success in mathematics at both Year levels, [Year 7 co-ed girls demonstrating a much higher attribution to luck: [80% v 39%], and Year 11 girls demonstrating a considerably higher attribution:[50% v 30%]; and, thirdly, the factor which contradicted the rest of my results, that of grasping new ideas quickly, which indicated that at Year 7 level the co-ed girls thought they had grasped the new ideas more quickly than their single-sex counterparts [100% v 66%], and at Year 11 level the figures were 52% v 33% in favour of the co-ed girls group. Belief in ability showed variation at Year 11 level only, where 58% of single-sex girls answered positively as did 37% of co-ed girls. These figures were supported by questions relating to confidence, enjoyment of trying to solve a new maths problem, and assumption of knowing the right answer. Finally, the last notable difference was the polarization towards gendered make-up of the class where 80% of single-sex girls thought single-sex education was preferable and 60% of co-ed girls thought co-education was preferable at Year 7 level. The figures increased at Year 11 level where 95% of single-sex girls preferred a single-sex setting and 81% of co-ed girls preferred co-education. Results which showed very little difference between the groups were attribution of hard work for success, importance of mathematics for careers, retention of mathematics as a part of a job, importance of mathematics for each sex, distribution of teacher attention and distribution of natural ability between the sexes.
In this sample, single-sex education seems to be more advantageous than co-education for girls. As international data on this topic varies according to the source, it is very difficult to relate it to these results. It does, however, provide evidence of some of the significant strides that have been made towards achieving gender equity, especially concerning girls' own thinking about the importance of mathematics, their ability, and the attribution of hard work for success.
The second group of comparisons is between single-sex girls and co-ed boys. Within my sample, these two groups generated some very interesting data as they provided evidence, at times, of almost identical thinking. Included in these almost identical responses are the attribution of hard work for success [particularly at Year 7 level], which indicates a significant improvement on many earlier studies; the belief that mathematics is required for successful employment; and the belief that mathematics would be an integral part of their job. This belief could have a positive influence on this girls' group pursuing mathematics to a higher level, which contradicts previous research indicating a general trend of able girls discontinuing their mathematical careers. A further identical factor was a belief that their respective mathematics teachers enjoyed teaching them. This factor could possibly be linked to self esteem as it could indicate a belief in self worth i.e. the teacher 'valued' their lessons.
The two groups produced identical results for the belief that mathematics is equally important for girls and boys and for the question reflecting teacher attention, both feeling they were asked as often as anyone else. At Year 11 level, the students thought they often knew the right answer when another student was questioned, which could indicate either a belief in confidence, in ability, or both. Their perception of hard work within the classroom was similar, too, and indicated a perception that most students worked hard most of the time at Year 7 level [70-80%] and that this had dropped off at Year 11 level in both instances [55% ssg 45% co-ed boys].
Factors which indicated differences in the thinking of these two groups included the following: belief in the distribution of natural ability between the sexes, where 41% of girls at Year 7 level thought that girls were more naturally able than boys, whereas 75% of the boys believed that mathematical ability was equally distributed. At Year 11 level, these trends reversed with 55% of boys believing that boys were naturally better at maths and 65% of single-sex girls believing natural ability to be equally distributed. At Year 11 level, only 30% of single-sex girls believed you have to be clever to do well at mathematics, whereas 61% of boys believed it. If these figures are linked to the importance of mathematics for each sex and the attribution of hard work for success, it would indicate very positive feelings by the single-sex girls - that they are prepared to work hard for the success which they consider important.
At Year 11 level, the co-ed boys had a more positive belief [74% v 48%] that their teacher thought that they were good at mathematics. It would be interesting to measure this statistic against attainment to see how it relates to confidence in order to ascertain whether there was in fact, a difference in ability or in confidence that produced these numbers. The comparative figures for confidence within this age level indicated a difference [58% single-sex girls v 74% co-ed boys] and reveal a substantial, but not as large a difference as that attributed to their teachers' belief.
Boys at both year levels indicated more enjoyment at trying to solve a new maths problem but this was only a 10 - 12% difference. At Year 11 level, only 16% of boys minded missing a maths lesson, compared with 45% of girls, which could be an indication of confidence or a lack of responsibility as previously mentioned.
A final major difference was the students' perception of whether own-sex only or co-ed was a more successful learning environment. At Year 11 level, the numbers indicate a dramatic difference where 95% of girls preferred single-sex schooling and 78% of boys preferred co-education. Whilst these figures indicate positive feelings for their own situation, they are speculative as the vast majority of the students involved have only experienced one teaching environment.
In very general terms these two groups demonstrated the most similarities.
My third group of comparisons is between the co-ed girls group and the co-ed boys group. These two groups indicated the greatest differences within the test samples and many of the factors showing differences could be directly or indirectly related to confidence.
Enjoyment of mathematics showed a 33% [ssg] and 72% [co-ed boys] difference at Year 11 level, a much larger difference than the Year 7 figures which were 100% v 85% where the girls, in fact, had the higher percentage. These figures are perhaps the most significant ones generated by my study.
Although both groups at each level attributed hard work to success, somewhat contradictory figures for question 4 indicated that 80% v 10% [Year 7] and 59% v 23% [Year 11] attributed success to luck. This is indicative of tremendous gender differences with my co-education sample. This lack of confidence is supported by the Year 11 response to question 10 which indicates that only 37% of girls compared to 74% of boys believed that they were good at maths. As mentioned previously, it would be very interesting to be able to link this to data on attainment, which was unavailable.
The younger groups displayed similar figures for grasping new ideas quickly, enjoying the challenge of new work, feeling confident in ability, whereas the Year 11 group showed much greater differences, in favour of boys, for these questions.
Both groups agreed on the importance of mathematics for both sexes, but only 39% thought that natural ability was evenly distributed between the sexes. 55% of them thought that boys had more natural ability at Year 7 level, whereas 56% of girls thought natural ability was equally distributed at Year 11 level. Year 7 figures both indicated a belief that ability was equally distributed; [80% +75%]. Question 21, indicating the perception of distribution of teacher attention, revealed interesting data; at year 7 level, 60% of girls perceived they received less teacher attention compared to 25% of boys, and at Year 11 level 45% of girls had this perception compared to 9% of boys. The overwhelming majority of boys [82%] thought that teacher attention was equally distributed between the sexes. These figures reinforce one of the negative implications of co-education.
At Year 11 level only 45% of co-ed students had the perception that most people worked hard most of the time. These were the lowest figures in the sample and also provide somewhat negative feedback for co-education.
Encouraging statistics were obtained from questions relating to the importance of mathematics for each sex [100%, 95% for Year 7, 81%, 90% for year 11] and the importance of mathematics within the job [80%, 85% for Year 7, 63%, 68% for Year 11].
Links with Other Research
Extensive research which highlights the belief that co-education per se does not achieve parity for female students or equity in policy or practice has caused much experimentation for girls in single-sex settings e.g. Smith [1986], and Sampson [1989]. These etudies with others suggest that well organized, carefully-timed, single-sex classes may lead to qualitative [i.e. attitudinal] if not quantitative [i.e. achievement] benefits in the learning of mathematics for at least some females. These studies have also highlighted subtle factors - time tabling and textbook choice for example, which disadvantage girls in a co-educational setting. Only in recent years have studies which examine the apparent benefits or disadvantages of long-term education in a gender-segregated environment begun to control for socio-economic factors i.e. single-sex students tend to come from higher socio-economic backgrounds.
The benefits and disadvantages of co-educational and segregated education need careful scrutiny. Contradictory research evidence does not support the adoption of long-term segregated mathematics classes. The A.P.U. [1982], Cockcroft [1982], Sampson [1989] and Smith [1986] have argued that girls studying mathematics seem to be disadvantaged by co-education. On the other hand, Bone [1983], Dale [1974], and Smith [1987] reported that girls in co-educational settings perform at least as well as those in single-sex schools. As mentioned previously, the work of Smith [1987] is particularly interesting which suggests that girls benefit from segregated mathematics teaching during the first two years of high school, followed by co-education during the next two years. Lee and Bryk [1986] found that boys performed better in mathematics, irrespective of the type of school attended; however, girls in single-sex schools were more likely to express an interest in mathematics and took more mathematics courses than those attending co-educational school. Rowe, [1988] in a two year case study within a co-educational school reported that the greatest gains in mathematics achievement were made by girls in single-sex classes.
Leder [1990] has acknowledged the difficulties in drawing comparisons across different school systems where equipment, staffing, class size and other variables may differ substantially. The influence of these variables on mathematics achievement is confirmed by Cresswell and Gubb [1987] in their analysis of data from the SIMS.
It is worth repeating that, in both England and Australia, significant sections of the population are educated in single-sex schools and the children are drawn from higher socio-economic homes. This point is acknowledged by Carpenter [1985].
In terms of my small sample, I feel that I gathered illuminative data on certain questions which enhanced my own belief in the benefits of single-sex teaching. There was evidence of some of the negative aspects of co-education, such as lack of teacher attention for girls, which were not present in the all-girls school. As stated previously, I think the most significant data produced by my study were the results of the questions pertaining to enjoyment, which demonstrated, in this instance, very positive implications for single-sex schooling in mathematics education.
Weaknesses of Study
I feel that the main weakness of my study was the limited area in which my research was performed. The student population on an island as small and as isolated as Bermuda is unique and atypical both socially and racially. My choice of schools was also very restricted and resulted in the use of two schools that may not have been ideally comparable. A further difficulty was a general resistance to releasing achievement data, possibly caused by the insular location of Bermuda, producing exaggerated rivalry between schools.
Further Questions Raised for Research
As an extension of this study, it would be interesting to tie attainment to the affective variables and monitor future mathematical success. This could include the pursuit of higher mathematical courses. A different aspect of this research would be to find the optimum possible combination of single-sex and co-education in terms of positive student attitude towards mathematics.
Appendix 1. Questionnaire
Student Questionnaire - Mathematics.
Please read the following statements carefully. Tick those which you agree with and put a cross against those you disagree with.
1] [] I enjoy maths.
2) [] You have to be clever to do well at maths.
3) [] You have to work hard to do well at maths.
4) [] I am lucky when I do well on a maths test.
5) [] I usually understand a new maths idea quickly.
6) [] I think that my maths teacher thinks that I work hard
at maths.
7) [] I think that my maths teacher thinks that I am good at
maths.
8) [] Knowing maths will help me get a job.
9) [] I think maths will be an important part of my job when
I leave school.
10)[] I believe that I am good at maths.
11)[] I enjoy trying to solve a new maths problem.
12)[] I feel confident about my ability in maths.
13)[] I do not like it if I miss a maths lesson.
14)[] I think my maths teacher enjoys teaching me.
Read the following statements, and tick the explanation which you feel applies to you.
15) If I do well in maths it is usually because:
[] I am naturally good at it.
[] I work very hard.
[] I was lucky.
[] The work is very easy.
16) If I do badly in maths it is usually because:
[] I am not naturally good at it.
[] I did not work hard enough.
[] I was unlucky.
[] The work is too hard.
17) To be good at maths is:
[] More important for girls.
[] More important for boys.
[] Equally important for boys and girls.
18) The teacher usually asks me to answer:
[] As often as anyone else.
[] More than anyone else.
[] Less than anyone else.
19) When the teacher asks another student to answer:
[] I usually know the right answer.
[] I sometimes know the right answer.
[] I hardly ever know the right answer.
20) In lessons most people work hard:
[] All of the time.
[] Most of the time.
[] Some waste too much time.
21) Do you feel you have the teacher's attention:
[] More than most other students.
[] Less than most other students.
[] The teacher's attention is equally distributed.
22) Do you think you would learn more if your class was:
[] Boys and girls together.
[] Just your own sex.
23) Which sex do you think is naturally better at maths?:
[] Boys
[] Girls
[] Both sexes do equally well.
24) Do you think it is more important for:
[] Boys to be good at maths?
[] Girls to be good at maths?
[] It is equally important for both.
25) Please tick: [] Girl. [] Boy.
Thank you very much for your assistance in completing this questionnaire.
APU [1981] Secondary Survey Report no.2, HMSO.
APU [1982] Mathematical Development, Secondary Survey [Report No 3]. London: HMSO.
APU [1988] Attitudes and Gender Difference, NFER-Nelson.
Armstrong, J. [1985] A national assessment of participation and achievement of women in mathematics. In S. Chipman, L.Brush and D. Wilson [eds.], Women and Mathematics: Balancing the equation [pp 59-94]. Hillsdale, NJ: Erlbaum.
Arnot, M. [1983] A Cloud over Co-education: An Analysis of the forms of Transmission of Class and Gender Relation. Open University. The Falmer Press: Sussex.
Barnes, M. [1991] An Introduction into Investigating Change in Australian Schools. Melbourne, Curriculum Corporation.
Barnes, M., Plaister, R. and Thomas, A. [1984] Girls count in Math and Science, for the project 'Increasing the participation of girls in mathematics'. Commonwealth Schools' Commission, Sydney, Australia.
Barsky, D., Chaleyat-Maurel, M., Christol, G., Godbillon, C., Klein, E., Merindol, J-Y., Moeglin, C., Ovaert, J-L., Raoult, A. Roussignol, M. and Simon, J. [1987] 'DŽmographie des mathŽmaticiens. Des mathŽmatiques a l'industrie. La Fuite des cervaux', Bulletin de la SocietŽ MathŽmatique de France. Supplement au tome 115, pp. 343-91.
Belenky, M.F., Clinchy, B.M., Goldberger, N.R. and Tarule, J.M. [1986] Women's Ways of Knowing: The Development of Self, Voice and Mind, New York, Basic Books.
Beloff, H. [1992] In Times Higher Educational Supplement, 17th April [Report by Sian Griffiths].
Benbow, C. and Stanley, J. [1983] Differential course-taking hypotheses revisited. American Educational Research Journal,20, 469-473.
Bone, A. [1983] Girls and Girls Only Schools: A Review of the Evidence [Equal Opportunities Commission].
Brophy, J., and Good, T. L. [1974] Teacher-student relationships: Causes and Consequences. New York: Holt, Rinehart and Winson.
Burton, L. [ed] [1990] Gender and Mathematics. An International Perspective, London, Cassell.
Buxton, T. D. [1982] Being and Becoming: Teachers' perceptions of sex-roles, and actions towards their male and female pupils, British Journal of Sociology of Education, vol.3 no.2, p 127-43.
Carpenter, P. [1985]. Single-sex schooling and girl's academic achievement. Australian and New Zealand Journal of Sociology, 21, 456-472.
Cockcroft, W.H. [1982]. Mathematics Counts, London: HMSO.
Cohen, L. and Manion, L.[1992] Research Methods in Education. Routledge: London.
Connell, R. W. [1987] Gender and Power, Cambridge, Polity Press.
Cresswell, M. & Gubb, J. [1987] The Second International Mathematics Study in England and Wales. Windsor, Berkshire: NFER-Nelson.
Cronkite, R. and Perl, T. [1978] 'Evaluating the impact of an intervention program: Math-science career conferences for young women' in Proceedings of the Conference on the Problem of Math Anxiety. Sponsored by the School of Natural Sciences, California State University, Fresno.
Dale, P, R. [1969, 1971, 1974] Mixed or single-sex school? Routledge and Kegan Paul: London.
Damarin, S. K. [1995] Gender and mathematics from a feminist standpoint. In New directions for equity in mathematics education. Secada, Fennema and Byrd, England: Cambridge University Press.
Defoe, D. [1697/1969] An essay upon projects. [A Scholar facsimile.] Menston, England: Scholar Press.
Delon, F. [1995] in Rogers,P. and Kaiser, G.[As below] The French Experience: The Effects of Desgregation, p 141-146.
Department of Education and Science. [1989] National Curriculum: From Policy to Practice [London DES]
Department of Employment, Education and Training. [1990]. Women and work, 12[3]. Canberra, Australia: Author.
Dunne, M. and Johnston, J. [1992] An awareness of epistemological assumptions: the case of gender studies. International Journal of Science Education, 14, 5 p.515-526.
Durham, M. [1985] Oxford goes for Compromise, The T.E.S. 20 Dec, p 6.
Dupe, M.-O. [Ed] [1992] Bizutages, Conde-sur-Noireau, Arlea-Corlet-Panoramique, n 6.
Dusek, J.B. and Joseph, G. [1985]. Teacher Expectancies. Hillsdale, NJ: Lawrence Erlbaum.
Dweck, C.S., Davidson, W., Nelson, S. and Enna, B. [1978] Sex differences in Learned Helplessness. Part 2: The Contingencies of Evaluative Feedback in the Classroom. Part 3: An Experimental Analysis. Development Psychology, 48, 268-276.
Eccles, J. [1983]. Expectancies, values and academic behaviours. In J.T. Spence [Ed.], Achievement and achievement motives [pp.75-146]. San Francisco: Freeman.
Educational Testing Service. [1988]. Profiles of SAT and achievement test takers. Princeton, NJ: Educational Testing Service.
Eichler, M. [1988]. Nonsexist research methods. Boston: Unwin Hyman.
Ernest, P. [1994]. Educational Research, It's Philosophy and Purpose. An introduction to Research Methodology and Paradigms. Exeter University Press.
Fennema, E. [1980] 'Sex-related differences in mathematical achievement: where and why' in Fox, L., and Brody, L. and Tobin, D. [eds] [1980] Women and the mathematical mystique, Johns Hopkins University Press.
Fennema, E. and Leder, G.C. [eds.]. [1990] Mathematics and gender. New York" Teachers College Press.
Fennema, E. and Peterson, P.L. [1985] Automonous learning behaviour: A possible explanation of gender-related differences in classroom interactions. In L. C. Wilkerson and C.B. Marrett [eds] Gender-related differences in classroom interaction [pp. 17-35]. New York: Academic.
Fennema, E. and Peterson, P. [1987]. Effective teaching for girls and boys: The same or different? In D. Berliner and B. Rosenshine [eds.], Talks to teachers [pp.111 - 25]. New York: Random House.
Fennema, E., Peterson, P., Carpenter, T. P. and Lubinski, C.[1991] Teacher's attributions and beliefs about girls, boys and mathematics. Educational Studies in Mathematics.
Fennema, E. and Sherman, J. [1976]. Fennema - Sherman Mathematics Attitude Scales. JSAS: Catalog of Selected Documents in Psychology, 6[1], 31. [Ms. No 1225]
Fennema, E. and Sherman, J. [1978]. Sex-related differences in mathematics achievement and related factors: A further study. Journal for Research in Mathematics Education, 9 [3], 189 - 203.
Fennema, E. and Sherman, J. [1979]. Sex-related differences in mathematics achievement , spatial-visualization and affective factors. American Educational Research Journal, 14, 51-71.
Fennema, E., Wolleat, P. and Pedro, J. D. [1979]. Mathematics Attribution Scale. JSAS: Catalog of Selected Documents in Psychology, 9 [5], 26. [Ms. No. 1837].
Forbes, S.D. and Mako, C. [1993] 'Assessment in Education: A Diagnostic Tool or a Barrier to Progress'. Paper presented at the 1993 World Indigenous People Conference: Education, Wollongong, Australia.
Fox, L.H., Fennema, E. , and Sherman, J. [eds] [1977]. Women and mathematics: Research perspectives for change. NIE Papers in Education and Work, No. 8. Washington, DC: United States Department of Health, Education, and Welfare.
Friedman, L. [1989]. Mathematics and the gender-gap: A meta-analysis of recent studies on sex differences in mathematical tasks. Review of Educational Research, 59 [2], 185 - 213.
GEMS [1990] Canberra, Curriculum Development Centre,1. p.1-5
Good, T. L. and Findley, M. J. [1985] Sex role expectations and achievement. In J. B. Dusek [Ed.], Teacher Expectancies [p.271-302]. Hillsdale, NJ: Lawrence Erlbaum.
Gore, D. A. and Roumagoux, D.V. [1983]. Wait time as a variable in sex -related differences during fourth grade mathematics instruction. Journal of Educational Research, 26, 273 - 275.
Gross, S. [1988]. Participation and performance of women and minorities in mathematics [vol. 1]. Rockville, MD: Montgomery Public Schools, Carver Education Services Centre.
Grosz, E. [1990] Conclusion: a note on essentialism and difference, in GUNEW, S. [ed] Feminist Knowledge. Critique and Construct, London, Routledge.
Grundy, S. [1987] Curriculum: Product or Praxis, London, The Falmer Press.
Habermas, J. [1972] Knowledge and Human Interests, 2nd edition, London, Heinemann.
Hanna, G. [1989] 'Mathematics achievement of girls and boys in grade eight: Results from twenty countries'. Educational studies, 20, pp.225-32.
Hartsock, N. [1983] The feminist standpoint: Developing the ground for a specifically feminist historical materialism. In S. Harding and M. Hintikka [eds.],Discovering reality: Feminist perspectives on epistemology, metaphysics, methodolgy, and philosophy of science [pp. 283-310]. Dordrecht, Holland: Reidel.
Hiddleston, P. [1995] The Contribution of Girls-only Schools to Mathematics and Science Education in Malawi. In Rogers, P. and Kaiser, G. [As below].
Hitchcock, G. and Hughes, D. [1992] Research and the Teacher Routledge, London
Hoiland, E. A. [1973] Interpretation of English Idioms by Indians and Non-Indians. Unpublished Master's Thesis. University of Saskatchewan, Canada.
Horner. M. S. [1986]. Sex differences in achievement motivation and performance in competitive and non - competitive situations. Unpublished doctoral dissertation, University of Michigan, Ann Arbor.
Hyde, J., Fennema, E., Ryan, M., Frost, L. and Hopp, C. [1990]. Gender comparisons of mathematics attitudes and affect: A meta - analysis. Psychology of Women Quarterly, 14 [3], 299 - 324.
Imbert, F. [1994] 'Agregations scientifiques et ENS au temps de la mixitŽ, Unpublished manuscript.
Jiminez, E., and Lockhead, M.E. [1989] The Relative Effectiveness of Single Sex Schools in Thailand, Education and Policy Analysis.
Johnson, D. D. [1973-74] Sex Differences in Reading across Cultures. Reading Research Quarterly, 9:67-86.
Kaeley, G.S. [1988] 'Sex Differences in the Learning of Post-secondary Mathematics in a Neo-literate society'. Educational Studies in Mathematics, 19 pp.435-57.
Kenway, J. and Willis, S. [1993] Telling Tales: Girls and Schools Changing Their Ways, Canberra, Department of Employment, Education and Training.
Lather, P. [1992] Critical frames in educational research: feminist and post-structural perspectives, Theory into Practice, 31,2,p.87-89.
Lawson, J. and Silver, H. [1973]. A social history of education in England. London: Methuen.
Leder, G.C. [1982]. Mathematics achievement and fear of success. Journal for Research in Mathematics Education, 13,[2], 124 - 135.
Leder, G.C. [1987]. Teacher-student interaction: A case study. Educational Studies in Mathematics, 18, 255 - 271.
Leder, G.C. [1990a] Gender differencesss in mathematics. An overview. In E. Fennema & G.C. Leder [eds.], Mathematics and gender [p10-26], New York: Teacher's College Press.
Leder, G.C. [1992]. Mathematics and gender: Changing perspectives. In D. A. Grouws [ed.], Handbook of research on mathematics teaching and learning [597 - 622].
Lee, V.E. and Bryk, A.S. [1986]. Effects of single-sex secondary schools on student achievement and attitudes. Journal of Educational Psychology, 78, p381-395.
Lee, V.E. and Lockhead, M.E. [1990] 'The Effects of Single-sex Schooling on Achievement and Attitudes in Nigeria'. Comparative Education Review, 34, 2. pp. 209-31.
Linn, M.C. [1990]. Gender, mathematics and science: Trends and recommendations. Paper prepared for the summer institute for the Council of Chief State Schools Officers, Mystic, CT.
Linn, M.C., and Hyde, J.S. [1989]. Gender, mathematics and science. Educational Researcher, 18, 22-27.
Lock, R. [1992] Gender and practical skill performance in science. Journal of Research in Science Teaching, 29, 3, p.227-41.
Macoby, E.E. and Jacklin, C.N. [1974], The Psychology of Sex Differences, Stanford: Stanford University Press.
Mahoney, P. [1985] Explorations in feminism: Schools for the Boys: Co-education Reassessed, Hutchinson: London.
Marland, M. [1983] Sex Differentiation and Schooling. Heinemann Educational Books Ltd: London.
Maritim, E.E. [1985] 'The Dependence of 'O' and 'A' level Results on the Sex of the Examinees'. Kenya Journal of Education, 2. pp 21-46.
Marston, M. [1995] Is there Equity for Boys? TES, November 1995.
Martini, R. [1982] Sex Differences and Achievement, ILEA Reasearch and Statistics, R.S. 823\82.
McLeod, D.B. [1988] Affective issues in mathematical problem-solving. Some theoretical considerations. Journal for Research in Mathematics Education, 19, 134-141
Measor, L. and Woods, P. [1984] Changing Schools, Milton Keynes: Open University Press.
Morrow, C. and Morrow, J. [1991, October] The SummerMath Programme for Minority Girls and Teachers, Annual Report, National Aeronautics and Space Administration Project.
Mura, R. [1991] Searching for Subjectiviyy in the World of the Sciences: Feminist Viewpoints, The CRIAW Papers, 25, Ottawa, Canadian Research Institute for the Advancement of Women.
National Education Goals Report: Building a Nation of Learners [1997] News Bulletin NCTM January 1998, 34 6.
National Science Foundation. [1990]. Women and minorities in science and engineering. Washington, DC: Author.
Open University. [1986] Girls into Mathematics, Press Syndicate of the University of Cambridge, Cambridge.
Ormerod, M. D. [1975] Subject Preference and Choice in Co-educational and Single-sex Secondary Schools, British Journal of Educational Psychology, 45 p 256-267.
Plato. [1955]. The Republic. [H.D.P. Lee, Trans.] New York: Penguin.
Powell, R.C. and Batters, J. [1986] Sex of teacher and the image of foreign languages in schools, Educational Studies, 12 p 245-254.
Reston, VA: National Council of Teachers of Mathematics and Science. New York: McMillan.
Reyes, L.H. [1984]. Affective variables and mathematics education. Elementary School Journal, 18 [2], 207 - 218.
Reyes, L.H., and Stanic, G.M.A. [1988]. Race,sex, socioeconomic status and mathematics. Journal for Research on Mathematics Education, 9[1], 26-43.
Rogers, P. [1990]. Thoughts on power and pedagogy. In L. Burton [Ed.], Gender and mathematics: An international perspective [38 - 46]. London: Cassell.
Rogers, P. and Kaiser, G. [1995] Equity in Mathematics Education: Influences of Feminism and Culture. London: Falmer Press.
Romberg, T.A. and Carpenter, T.P. [1986]. Research on teaching and learning mathematics: Two disciplines of scientific inquiry. In M.C. Wittrock [Ed.], Third handbook of research and teaching [850 - 873]. New York: McMillan.
Rowe, K. J. [1988], Single-sex and mixed-sex classes: The effects of class type on student achievement, confidence and participation in mathematics classes. Australian Journal of Education, 32, 180-202.
Ruskai, M.B. [1990]. Why women are discouraged from becoming scientists. Scientist March 5, 1990 p17+.
Sampson, S. [1989]. Are boys a barrier for girls in science? In G.C. Leder & S.N. Sampson [eds.], Educating girls, Practice and Research, [p 139-143]. Sydney: Allen and Unwin.
Secada, W.G. Fennema, E. and Adajian L.B. [1995] New Directions foe Equity in Mathematics Education, Cambridge University Press: Cambridge.
Shaikh, S. and Kelly, A. [1989] To mix or not to mix?, Educational Research, 31 p 10-19.
SIMS, [1981] In 'Equity in Mathematics Education' p 109, [see Rogers, P. and Kaiser, G.]
Sherman, J. [1983] Girls talk about mathematics and their future: A partial replication, Psychology of Women.
Smith, S. [1986] Separate Tables? An Investigation into Single-sex Setting in Math. London: HMSO.
Smith, S. [1987] Separate beginnings? Manchester Equal Opportunities Commission.
Squire, C. [1989]. Significant differences: Feminism in psychology. New York: Routledge.
Stables, A. [1990] Differences between Pupils from Mixed and Single-sex Schools in their Enjoyment of School Subjects and in their Attitudes to Science and School: Educational Review, Vol 42, no 3, Carfax Publishers.
Steedman, J. [1983] Examination Results in Mixed and Single-sex Schools: Findings from the National Child Development Study. [Equal Opportunities Commission]
Sutherland, M. B. [1985] Whatever Happened about Co-education? British Journal of Educational Studies, 33 p 155-163.
Tobias, S. [1978]. Overcoming math anxiety. Boston: Houghton Mifflin.
Turkle, S. [1984]. The second self:Computers and the Human spirit. New York: Simon and Schuster.
Veroff, J. [1977] Process vs impact in Men's and Women's Achievement Motivation. Psychology of Women Quarterly. 1:283-293.
Waldern , R. and Walkerdine, V. [1985] Girls and Mathematics: From Primary to Secondary Schooling, Bedford Way Papers 24, London, Heinemann.
Webb, N.M. [1984] 'Sex differences in interaction and achievement in cooperative small groups', Journal of Educational Psychology, 76, p 33-44.
Webb, N.M. and Kenderski, M. [1985] Peer Interaction and Learning in Small Groups, 13[1], 21-39.
Weinekamp, H. et al [1987] Does unconscious behaviour of teachers cause chemistry lessons to be unpopular with girls?, International Journal of Science Education, 9, p 281-286.
Whitehorn, K. [1984] How to Teach the Young. Observer Magazine, 14 October, p 31-32.
Whyte, J. [1986] Girls into Science and Technology: The Story of a Project. London. Routledge and Kegan Paul.
Wilce, H. [1986] ILEA exam study backs girls' schools, The Times Educational Supplement, 3 Jan, p 1-3.
Willis, S. [1989] Real Girls Don't Do Maths, The Construction of Priviledge, Geelong, Deakin University Press.
Willis, S. [1995] Gender Reform Through School Mathematics. in Rogers, P. and Kaiser, G.[1995] [see above].
Woods,P. and Hammersley, M. (1993) Gender and Ethnicity in schools. Ethnographic Accounts. New York: Routledge.
World Bank [1988] Education in Sub-Sahara Africa. Policies for Adjustment, Revitalisation, and Expansion, Washington, DC, World Bank.