
Luqian Zhou
<luqianzhou@hotmail.com>
@ is replaced by (at) to stop the automatic garnering of email addresses by Spam factories - Editor
Introduction
Pearson (1989) suggests that teaching is intended to create learning, and this seems a reasonable starting position. Mathematics teaching is intended to create the learning of mathematics1 . Over a century an impressive array of researchers has been contributing to the effective teaching methodology relating to children's learning of mathematics. The focus of many studies has shifted from the instructivist approach to the constructivist approach, highlighting learning is seen in terms of the student or the class constructing mathematical ideas for themselves.� Constructivism encourages educators to recognise their students' strongly held preconceptions and to provide experiences that will help them build on their current knowledge of the world (Duit & Confrey, 1996). 2
What is mathematics teaching? What is the best way to teach?
Mathematics educators from all over the world are
delving deeply into the desirable teaching strategies, which engage students
with the cognitive demand and thus result in more widely effective mathematical
learning.� The whole-class interactive teaching method recommended by The Mathematics
Enhan cement Project (MEP) which was developed by the Centre for Innovative
in Mathematics Teaching (CIMT) at the University of Exeter has shaped or coloured
teachers' new educational experience in mathematics classrooms in stark contrast
to the traditional teaching methods adopted currently.
The Numeracy Task Force (DfEE 1998) has placed some emphasis on whole class teaching.
"Inspection evidence and the experience of the National Numeracy Project point to an association between more successful teaching of numeracy and a higher proportion of whole class teaching. " (para 41 p19)
They also go on to assert:
"Some of the countries that do best in international comparisons, such as Japan and Korea, report a high frequency of lessons in which children work together as a class, and respond to one another." (para 42 p19)
In the Summary of recommendations, the Task Force include:
"devoting more time in mathematics lessons to direct communication with pupils, particularly by teaching the whole class together" (p 2) 3
Review of my teaching in China and the UK
M athematics education in different countries is strongly
influenced by cultural and social factors that build goals, beliefs, expectations,
and teaching methods.� Different cultures and societies have different philosophies
regarding the teaching and learning of mathematics.� I 'm lucky to experience
teaching in China and the UK and witness the different teaching styles and strategies,
which have a great impact on children's learning of mathematics.
1. Interactive W hole Class Teaching in China
1) Class Size and the Scheme of Work
The school I had been teaching in for three years is a key
primary school in Suzhou.� In contrast to the set system in the UK, Chinese
students learn the same topic using the same textbooks at all times.� While
UK classes are often no more than 25-30 students, in China many classrooms have
45-60 students Large class sizes necessarily mean
less flexibility in room arrangements, and thus most Chinese classrooms have
students sharing small desks and sitting in rows. The class I had comprised
52 students. I had been teaching them from Y1 to Y3, following a more
uniform and systematic curriculum. At the beginning of each term, we had the
district mathematics team meeting in which involved all the maths teachers in
the same area. We discussed the difficult teaching points and exchanged
the teaching strategies and ideas of a particular topic. At the same time,
we were given the uniform scheme of work for the whole term since all schools
used the same textbook. This scheme of work consisted of the strict teaching
hours and the date for each mathematics topic that arranged systematically and
in depth. It also pointed out the date for exams and holidays gave two
to three flexible lessons for teachers in case some teachers couldn't complete
the teaching task due to school activities. After we got the scheme of work
and teacher's reference book, which stated the objectives and some recommended
teaching ideas of each lesson; we started to plan every lesson c arefully considering
the possible learning difficulties the students might have.
2) Four-step Teaching Procedure
My students had one 45-minute maths lesson every day.
Maths teaching in my school basically followed the teaching method that we called
Fou r-Step Teaching Procedure.
Revision It's very important
to revise the main point learned in previous lessons. In Year 1 and Year
2, we focused on arithmetic. Number cards involving addition, subtraction,
multiplication and division were my starting poi nt of every lesson. To
speed up the children's mental calculation was the main task for us. I
used various activities, such as Driving My Train , Maths Relay , and etc .
in which students stood up and give answers one after another. A star
or a red flowe r was given as the praise to the fast groups. On one hand,
my students were motivated because they loved competition. On the other
hand, they practised their mental calculation every day. At the end of
Year 1, the students had oral and written tests on one and two digit number
arithmetic calculation. In my school all students must get no more than
two errors in 60 questions and the time limit was less than three minutes.
In 1998 and 1999, I had two students who completed all 60 questions in only
52 seconds ! 95% of my students got all correct answers within two minutes.
The second teaching feature in my school was to revise the
relative skills and concepts needed for the new lesson. Therefore the
students had another opportunity to refresh the concepts they had learned and
could understand and construct the new skills and mathematical concepts easily.
Presentation This step can well
represent the striking interactive whole class teaching in Chinese classrooms.
It includes innovative elicitation for the new topic, the coherent presentation
strategies and the teacher-student dialogue that monitors the progress of learning.
I would like to use a specific example to illustrate this
step. The aim of the lesson was to analyse the quantitative relations
of the g iven information and solve the word problems. The activity I
used to introduce the new topic was asking my students to guess how many apples
in the bag. They had to ask me questions, such as Is it less than the
number of students in my group? Is it an even number? and etc. My students
were very curious to know the exact number of apples I had. They kept
asking questions and got to know that the information I gave had quantitative
relations of each other. I found the whole activity aroused their interest in
learning and the atmosphere created in the classroom was lively and exciting.
Then I presented the first word problem on the board.
The students in Red Star School were planting trees.
Year 3 had planted 36 trees. The number of the trees Year 4 had planted
was as twice as the trees Year 3 had planted. Year 5 had planted 8 trees
fewer than the total number of the trees of Year 3 and Year 4. How many trees
had Year 5 planted?
Can you draw some lines to present the information of this
problem? I asked my students to tell me what to draw first and how to present
the number of the trees Year 5 had planted. After their answers I showed
the following diagram on the board.

| Student A: 36+36x2-8 | Student B:36 x ( 2+1 ) -8 |
|---|---|
| =36+72-8 | =36*3-8 |
| =108-8 | =108-8 |
| =100 | =100 |
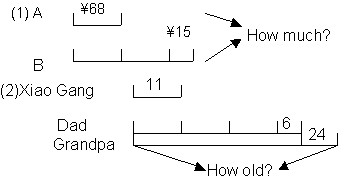
Summary & Homework
I always gave my students different kinds of questi ons to practise after the
new lesson. In China, students must complete all the class work and homework
assigned by teachers. Teachers
always give the work back the next day.
2. My First Year in the UK
To my great surprise maths teaching in the UK is very
different from that in China. During my first year in a mixed community
secondary school I noticed that there was a strong
investigational approach, group work dominated, calculators were used unrestrictedly.
My students tended to ask for help anytime in the lesson. Another noticeable
feature of the British maths teaching is that students are grouped in accordance
with their mathematical ability from Year 7. I believe the purpose of doing
so is to develop mathematical skills and knowledge at student s' own level and
learning pace. It's very essential to meet their individual needs.
However, many low ability students have lost their confidence from early years
in school because of the grouping and teachers' comments. Unconsciously
we mark those students as th e ones below our standard and give them more individualised
teaching and easy work, thinking that they cannot cope with the standard work.
Not surprisingly may we find that some Year 8 students can't even do the work
which should be learnt long time ago. Little by little, the ability gap
between able students and these low sets students is getting bigger and bigger.
A great number of students failed in maths learning or develop their growing
hatred towards this interesting and important subject. Should w
e ask ourselves, Is it partly due to our traditional teaching approach? Is that
what we expect from our good intention of meeting individual needs? David and
Clare Mills
4 pointed out that huge differences were discovered
in the way mathematics was taught in comparison with the students' academic
performance in Hungary, Germany, Switzerland, Flemish Belgium and the Pacific
Rim. It was found that successful countries invariably used interactive
whole class teaching rather than the individualised teaching the n current in
British schools.
How can we make the best learning of our students and narrow
down the gap by pushing our low ability students to meet our standard objectives?
Whole class interactive teaching studied in University of Exeter and the striking
test results of MEP schools has convinced us that we can change our teaching
routines to make learning meaningful, visible and applicable.
Looking back on the whole class teaching in other coutries,
Tibor Szalontai 5
enlightened us with the attractive ma in features of Hungarian teaching.
The question now put forward is how we can learn from other
successful countries and apply the essential parts of whole class interactive
te aching to our present mathematics education system. As all other mathematics
teachers, I believe an environment needs to be created through which all students
can have the opportunity to gain access to mathematics, learn mathematical skills,
develop an abi lity to apply mathematics in everyday circumstances and experience
joy in being able to do and understand mathematics .
MEP strategies reveal the essence of this method and recommend
the following. 6
1. Prepare everything before the lesson
2. Begin by reviewing homework
3. Warm up with mental arithmetic
4. Tell pupils the aim of the lesson
5. Give clear, precise instructions
6. Work through examples on the board interactively
7. Encourage as many pupils as possible to work at the board
8. Vary the questioning
9. Insist on mat hematical precision in oral and written work
10. Correct mistakes and misconceptions as they arise
11. Monitor the progress of every pupil
12. Vary the pace and activities
13. Use enthusiasm and humour
14. Praise pupils
15. Summarise the lesson
16. Set homework clearly ( shoul d be linked to the next lesson )
Is it possible
to combine my Chinese whole class teaching style and the MEP interactive teaching
strategies into my English class? I try to implement my whole class interactive
teaching in every lesson by reviewing home work. At first, my students were
not used to it as they had been used to the individual teaching style. But after
I constantly encouraged my students to work on the board, varied the pace and
activities and praised their good work, they started to like th e way I teach
and became very active in my lessons.
Application of Whole Class Interactive Teaching
Now I work in a Catholic boy school teaching across abilities
from 11 year olds to 16 year olds. The ethos of the department was different
from any I ha d yet experienced and I could see that it would be challenge to
adapt my mainly whole class interactive style to this new ethos. The lessons
I saw all had a similar structure. They started with an initial exposition then
the class would be set work, usually from a text book, and then the teacher
would walk round talking to the pupils individually.
However, I'm convinced myself that I can apply the
whole class interactive teaching in my class. Emphasis on understanding pupil
attainment linked with a willing ness to experiment with different styles of
teaching can lead to a steady growth in knowledge about teaching and learning
maths. (J. Ridgeway 1988) 7
I set myself some
teaching aims. One of them is to find ways to make maths less
daunting, give it meanin g and connect it with our real life.
The Numeracy Strategy would tell me what to teach but meanwhile I need to find
the link between each topic and find the best ways to avoid blunt knowledge
intake. Ridgeway described two main ways that new knowledge is f itted in with
existing thoughts and knowledge. The first of these is assimilation where the
way that new information is perceived is heavily dependent on present knowledge
and conceptual structures. Accommodation is when the new information is so differen
t from what was already understood that it forces the learner to change their
existing knowledge and conceptual structures. "To foster accommodation
it is necessary to provide dramatic examples which violate current concepts
and to provide these examples i n quantity." (Ridgeway 1988)
Major leaps in understanding come about by accommodation.
One way to bring this about would be if "Pupils are encouraged to make
mistakes and are seduced into errors which they reflect upon and remediate for
themselves or with the teachers help," (J. Ridgway, 1988). Backhouse, Haggarty,
Pirie & Stratton agree with Ridgeway and suggest that cognitive conflict
should be used to force learners to reject flawed methods. They also warn against
teaching that will only necessitate assi m ilation. "Beware of giving lots
of easy practice questions on which learners develop their own defective methods."
(Backhouse, Haggarty, Pirie, Stratton, 1992). The Shell Centre adds that as
well as being unproductive too many similar practice questions a r e boring
for both high and low attainers. 8
However I believe that teaching that only promotes one of
these two kinds of learning is flawed. If one only put pupils in situations
where the number of counter examples to what they would predict with misconce
ived methods is small then they might use this to refine their preconceived
ideas but they would never truly irradiate their misconceptions. However if
they are confronted constantly with situations that challenge their beliefs
it will be hard for any con c eptual structures to form. In the long run they
might be able to develop a misconception free understanding of the topic. Unfortunately
the high levels of confusion that would fill the interim period might stop them
from ever reaching that goal.
One must u se assimilation to allow correct new knowledge
to fit in with what the learner already knows. "Look for meaningful links
with other mathematics. This is important because relation learning is more
efficient than instrumental learning," 9
(Backhouse, Haggart y, Pirie, Stratton, 1992). I taught my
Year 8 students how to calculate the area of trapeziums. The topic that they
were on before it was areas of triangles and parallelograms. Thus revising how
to calculate areas of triangles and parallelograms first ena b les students
to link their present knowledge with the new topic. Learning is concerned
with making and strengthening links in their minds between new knowledge and
what they already know, 10
(Backhouse, Haggarty, Pirie, Stratton, 1992).
I started my les
son by asking , How many 2-D shapes have we learnt before? How can we calculate
areas of triangles and parallelograms? Then I presented some triangles and
parallelograms for my students to work out their areas. Next I presented a trapezium
on OHP and aske d if they could work it out as well. Some students looked puzzled
and I introduced the topic by hinting to convert the trapezium to the shapes
they had learnt before. I prepared some identical trapeziums and gave them out.
I asked my students to put them t ogether to see what kind of shape they could
make. One student came to the board and showed us that he could make a parallelogram.
My students were very interested in coming to the front and demonstrated their
shapes. Then I let them discuss in pairs what relations they could find between
a trapezium and a parallelogram.
The base of the parallelogram is equal to________. Its
height is equal to________. The area of each trapezium is_______ of the area
of the parallelogram we make.
Can you work out the f ormula to calculate the area of
a trapezium? My students could easily find out the formula after the discussion.
In order to consolidate this new knowledge, I continued to ask, What does
(base + top) represent? Why does it need to be divided by 2? When all my students
understood the formula and knew how to use it to work out the area of trapeziums,
I gave them some exercises to do and invited a couple of students to show their
working out on the board.
After a brief summary I extended the topic by aski ng my
students to think about whether they could use other ways to get the formula.
I changed my whole class teaching into group work. I divided my students into
groups of four and gave them some trapeziums and scissors. After fifteen minutes,
some groups worked out the new methods to find the formula. Then I demonstrated
the following ways on OHP.
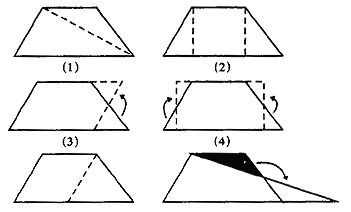
Each group explained how to use their own ways to find out
the formula. Finally I concluded that whatever methods we use, the formula of
area of trapezium is (base + top) � 2.
I moved to the textbook afterwards and set some more exercises
as consolidation. The whole lesson was very exciting and my students learnt
the new skills from their previous knowledge. Interestingly, I found the extension
was not so difficult as I thought because with interactive whole class teaching
and the varied activities, my students were encouraged to develop their mathematical
thinking and assimilated their new knowledge smoothly.
Conclusion
The core belief of British early years provision is that
children should move at their own, individual pace. In effect this always means
facilitating the faster progress of the more privileged and able. It is considered
inevitable that more privileged or more able children will move ahe a d of their
less advantaged peers. As a result, many children who feel themselves falling
behind begin withdrawing from the educational process. The difference gap has
become bigger and bigger. How to bridge the gap to push those less able students
to achi e ve higher in their maths learning? A great number of studies have
shown us that the adoption of interactive whole class teaching plays a major
role in transformation of the maths education. Yet anyone familiar with teaching
will recognise that any phrase such as 'interactive whole class teaching'
will have a multiplicity of interpretations in classrooms. Some might
be effective, some not, but the rhetoric itself will not ensure effectiveness.
I strongly hold that a successful maths teacher should have
beliefs about pupils, mathematics, teaching and the strategies he uses. It is
possible for us to enhance effective learning by providing the chance for more
interactions with more children in our daily class. On the other hand, whole
class interactive teaching strategy, I believe, doesn't rule out the
effective use of group work and other forms of learning. Some research indicated
that small-group work is more effective for higher cognitive skills like problem
solving. It convinced me in area of trapezium lesson I discussed above.
The teaching strategies recommended by MEP guide us towards the exploration
of successful maths teaching. This is a long road full of challenges. Perhaps
in a long term it might not prove as fruitful as it does in other successfu
l countries. However, more importantly, we must keep reflecting the way we teach
and how to teach interactively. It's not the literal understanding of
whole class interactive teaching but rather how you use it to enhance effective
learning which really see ms to count.
References
1. Jaworski , Barbara The Student-Teacher-Educator-Researcher in the Mathematics Classroom: Co-learning partnerships in mathematics teaching and teaching development University of Oxford ( Paper Presented at MADIF 2, January 2000 , Gothenburg, Sweden ) p.1
2. Duit R. & Confrey J. (1996). Reorganising the curriculum and teaching to improve learning in science and mathematics. In D.F. Treagust, R. Duit, & B.J. Fraser (Eds), Improving Teaching and Learning in Science and Mathematics. (pp. 79-93). New York and London: Teachers College Press.
3. Billington Eileen, Faculty of Education, University of the West of England, Bristol, & Fletcher Alison, Mangotsfield School, South Gloucestershire More Talk, Less Chalk? : An Exploration of Whole-Class Interactive Teaching in Mathematics (research paper from http://www.yahoo.co.uk, para1, p.1)
4. David and Clare Mills (June 2000) Lessons Britain Won't Learn Mills Productions Ltd., chapter3a,para 4
5. Tibor Szalontai(June 2000) Facts & Tendencies in Hungarian Maths Teaching (pp.1-2) http://www.ex.ac.uk/cimt/ijmtl/tshungmt.pdf
6. IPMA
conference report (Latimer,2002),CIMT University of Exeter
7-10. Rodgers , Alex : Curriculum development Algebra
year eight , s ection2c, (research paper from
http://www.yahoo.co.uk
)